Ký số [ sửa ]
Loại Ký số Biểu tượng số
Ký số La Mã
I
II
III
IV
V
VI
VII
VIII
IX
X
Ký số Ả rập
1
2
3
4
5
6
7
8
9
10
Ký số Trung quốc
-
=
Giá trị
1
2
3
4
5
6
7
8
9
10
Số đại số [ sửa ] Toán đại số dùng chữ cái a-z, A-Z đại diện cho các con số số học từ 0 đến 9. Thí dụ như A = 3 , B = 2 . Các chữ cái đại diện cho các con số số học được gọi là Biến số.
Loai số đại số [ sửa ] Số đại số được phân loai thành các loại số dưới đây
Số tự nhiên [ sửa ]
Loai số đại số Định nghỉa Ký hiệu Thí dụ
Số tự nhiên
N
{\displaystyle N}
0
,
1
,
2
,
3
,
4
,
5
,
6
,
7
,
8
,
9
{\displaystyle 0,1,2,3,4,5,6,7,8,9}
Số chẳn Mọi số chia hết cho 2
2
n
{\displaystyle 2n}
2
,
4
,
6
,
{\displaystyle 2,4,6,}
Số lẻ Mọi số không chia hết cho 2
2
n
+
1
{\displaystyle 2n+1}
1
,
3
,
5
,
7
,
9
{\displaystyle 1,3,5,7,9}
Số nguyên tố Mọi số chia hết cho 1 và cho chính nó
p
{\displaystyle p}
1
,
3
,
5
,
7
{\displaystyle 1,3,5,7}
Số nguyên [ sửa ] Số nguyên là số đại số bao gồm ba loại số số nguyên âm , số nguyên dương và số không . Số không có giá trị bằng 0 . Số nguyên âm có giá trị nhỏ hơn 0 . Số nguyên dương có giá trị lớn hơn 0Thí dụ như -1,0,+1
Số nguyên có ký hiệu chung
I
{\displaystyle I}
Số nguyên âm có ký hiệu chung
−
I
{\displaystyle -I}
Số nguyên dươngcó ký hiệu chung
+
I
{\displaystyle +I}
Số không
0
{\displaystyle 0}
Số nguyên âm .
−
1
,
−
2
,
.
.
.
−
9
{\displaystyle -1,-2,...-9}
Số nguyên dương .
+
1
,
+
2
,
.
.
.
+
9
{\displaystyle +1,+2,...+9}
Số nguyên không .
0
{\displaystyle 0}
Phân số [ sửa ] Số Phức là số có dạng tổng quát
a
b
{\displaystyle {\frac {a}{b}}}
Số thập phân
0
,
1234
{\displaystyle 0,1234}
Số hửu tỉ
3.1415...
{\displaystyle 3.1415...}
Số vô tỉ
0
,
33333....
=
1
3
{\displaystyle 0,33333....={\frac {1}{3}}}
Số Phức [ sửa ] Số Phức là số có dạng tổng quát
Z
=
a
±
j
b
{\displaystyle Z=a\pm jb}
Phép toán số đại số [ sửa ] Các phép toán đại số thực thi trên các số đại số bao gồm
Toán Ký Hiệu Công Thức Định Nghỉa
Toán cộng
+
{\displaystyle +}
A
+
B
{\displaystyle A+B}
Toán Cộng hai số đại số
Toán trừ
−
{\displaystyle -}
A
−
B
{\displaystyle A-B}
Toán Trừ hai số đại số
Toán nhân
x
{\displaystyle x}
A
×
B
{\displaystyle A\times B}
Toán Nhân hai số đại số
Toán chia
/
{\displaystyle /}
A
/
B
{\displaystyle A/B}
Toán Chia hai số đại số
Toán lũy thừa
a
n
{\displaystyle a^{n}}
a
n
=
a
×
a
×
a
.
.
.
{\displaystyle a^{n}=a\times a\times a...}
Toán tìm tích n lần của chính số nhân
Toán căn
{\displaystyle {\sqrt {}}}
a
=
b
{\displaystyle {\sqrt {a}}=b}
b
n
=
a
{\displaystyle b^{n}=a}
Toán lủy thừa nghịch
Toán log
L
o
g
,
L
n
{\displaystyle Log,Ln}
L
o
g
10
a
=
b
{\displaystyle Log_{10}a=b}
10
b
=
a
{\displaystyle 10^{b}=a}
Toán Toán lủy thừa nghịch của một lủy thừa
Phép toán Số nguyên [ sửa ] Số nguyên
I
=
I
<
0
,
I
=
0
,
I
>
0
{\displaystyle I={I<0,I=0,I>0}}
Phép toán Lũy thừa [ sửa ]
a
n
=
a
×
a
×
a
×
a
.
.
.
×
a
{\displaystyle a^{n}=a\times a\times a\times a...\times a}
Phép toán Toán căn [ sửa ]
n
a
=
b
{\displaystyle n{\sqrt {a}}=b}
a
=
b
n
{\displaystyle a=b^{n}}
Phép toán Toán log [ sửa ]
L
o
g
a
b
=
c
{\displaystyle Log_{a}b=c}
a
c
=
b
{\displaystyle a^{c}=b}
Toán Log Công thức
Viết tắc
L
o
g
=
L
o
g
10
{\displaystyle Log=Log_{10}}
L
n
=
L
o
g
2
{\displaystyle Ln=Log_{2}}
Log 1
L
o
g
(
1
)
=
0
{\displaystyle Log(1)=0}
Log lũy thừa
L
o
g
n
(
A
)
n
=
A
{\displaystyle Log_{n}(A)^{n}=A}
Lũy thừa log
B
L
o
g
B
(
A
)
=
A
{\displaystyle B^{Log_{B}(A)}=A}
Log của tích số
L
o
g
(
A
B
)
=
L
o
g
A
+
L
o
g
B
{\displaystyle Log(AB)=LogA+LogB}
Log của thương số
L
o
g
(
A
B
)
=
L
o
g
A
−
L
o
g
B
{\displaystyle Log({\frac {A}{B}})=LogA-LogB}
Log của lủy thừa
L
o
g
(
A
n
)
=
n
L
o
g
A
{\displaystyle Log(A^{n})=nLogA}
Đổi nền log
L
o
g
a
x
=
L
o
g
x
L
o
g
a
{\displaystyle Log_{a}x={\frac {Logx}{Loga}}}
Phép toán Toán số phức [ sửa ] Số phức được biểu diển như ở dưới đây
Số phức Thuận
Z
{\displaystyle Z}
Nghịch
Z
∗
{\displaystyle Z^{*}}
Biểu diển dưới dạng xy
Z
=
x
+
j
y
{\displaystyle Z=x+jy}
Z
=
x
−
j
y
{\displaystyle Z=x-jy}
Biểu diển dưới dạng Zθ
Z
∠
θ
=
x
2
+
y
2
∠
t
a
n
−
1
y
x
{\displaystyle Z\angle \theta ={\sqrt {x^{2}+y^{2}}}\angle tan^{-1}{\frac {y}{x}}}
Z
∠
θ
=
x
2
+
y
2
∠
−
t
a
n
−
1
y
x
{\displaystyle Z\angle \theta ={\sqrt {x^{2}+y^{2}}}\angle -tan^{-1}{\frac {y}{x}}}
Biểu diển dưới dạng hàm số lượng giác
Z
=
z
(
c
o
s
θ
+
j
s
i
n
θ
)
{\displaystyle Z=z(cos\theta +jsin\theta )}
Z
=
z
(
c
o
s
θ
−
j
s
i
n
θ
)
{\displaystyle Z=z(cos\theta -jsin\theta )}
Biểu diển dưới lũy thừa của e
Z
=
z
e
j
θ
{\displaystyle Z=ze^{j\theta }}
Z
=
z
e
−
j
θ
{\displaystyle Z=ze^{-j\theta }}
Toán số phức được thực thi như sau
Toán Số phức Toán cộng Toán trừ Toán nhân Toán chia
x
+
j
y
{\displaystyle x+jy}
x
−
j
y
{\displaystyle x-jy}
2
x
{\displaystyle 2x}
2
y
{\displaystyle 2y}
x
2
−
y
2
{\displaystyle x^{2}-y^{2}}
x
2
−
y
2
x
−
j
y
{\displaystyle {\frac {x^{2}-y^{2}}{x-jy}}}
x
+
j
y
{\displaystyle x+jy}
x
−
j
y
{\displaystyle x-jy}
2
x
{\displaystyle 2x}
2
y
{\displaystyle 2y}
x
2
−
y
2
{\displaystyle x^{2}-y^{2}}
x
2
−
y
2
x
−
j
y
{\displaystyle {\frac {x^{2}-y^{2}}{x-jy}}}
x
+
j
y
{\displaystyle x+jy}
x
−
j
y
{\displaystyle x-jy}
2
x
{\displaystyle 2x}
2
y
{\displaystyle 2y}
x
2
−
y
2
{\displaystyle x^{2}-y^{2}}
x
2
−
y
2
x
−
j
y
{\displaystyle {\frac {x^{2}-y^{2}}{x-jy}}}
Z
=
z
e
j
θ
{\displaystyle Z=ze^{j\theta }}
Z
=
z
e
−
j
θ
{\displaystyle Z=ze^{-j\theta }}
z
(
e
j
θ
+
e
−
j
θ
)
{\displaystyle z(e^{j\theta }+e^{-j\theta })}
z
(
e
j
θ
−
e
−
j
θ
)
{\displaystyle z(e^{j\theta }-e^{-j\theta })}
z
2
{\displaystyle z^{2}}
e
j
2
θ
{\displaystyle e^{j2\theta }}
Định lý Demoive
(
Z
∠
θ
)
n
=
Z
n
∠
n
θ
{\displaystyle (Z\angle \theta )^{n}=Z^{n}\angle n\theta }
Dải số đại số [ sửa ] Dải số [ sửa ] Dải Số là một chuổi số có định dạng . Thí dụ
Dải số của các số tự nhiên
1
,
2
,
3
,
4
,
5
,
.
.
.
.
,
n
{\displaystyle 1,2,3,4,5,....,n}
Dải số của các số tự nhiên chẳn
2
,
4
,
6
,
8
,
10
,
.
.
.
,
2
n
{\displaystyle 2,4,6,8,10,...,2n}
Dải số của các số tự nhiên lẻ
1
,
3
,
5
,
7
,
.
.
.
,
2
n
+
1
{\displaystyle 1,3,5,7,...,2n+1}
Tổng dải số đại số [ sửa ]
Chuổi sô Định nghỉa Ký hiệu Thí dụ
Chuổi số
phép toán tìm tổng của một dải số
S
=
∑
{\displaystyle S=\sum }
S
n
=
∑
i
=
1
n
a
i
=
1
+
2
+
⋯
+
n
=
1
+
2
+
3
+
.
.
.
+
n
=
k
(
1
+
n
)
{\displaystyle S_{n}=\sum _{i=1}^{n}a_{i}=1+2+\cdots +n=1+2+3+...+n=k(1+n)}
Tổng chuổi số cấp số cộng [ sửa ] Dạng tổng quát
a
+
(
a
+
d
)
+
(
a
+
2
d
)
+
.
.
.
+
[
a
+
(
n
−
1
)
d
]
=
∑
k
=
0
∞
[
a
+
(
n
−
1
)
d
]
{\displaystyle a+(a+d)+(a+2d)+...+[a+(n-1)d]=\sum _{k=0}^{\infty }[a+(n-1)d]}
Chứng minh
∑
k
=
0
∞
[
a
+
(
n
−
1
)
d
]
=
a
+
(
a
+
d
)
+
(
a
+
2
d
)
+
.
.
.
+
[
a
+
(
n
−
1
)
d
]
=
n
2
(
2
a
+
(
n
−
1
)
d
)
{\displaystyle \sum _{k=0}^{\infty }[a+(n-1)d]=a+(a+d)+(a+2d)+...+[a+(n-1)d]={\frac {n}{2}}(2a+(n-1)d)}
S
=
a
+
(
a
+
d
)
+
(
a
+
2
d
)
+
.
.
.
+
[
a
+
(
n
−
1
)
d
]
{\displaystyle S=a+(a+d)+(a+2d)+...+[a+(n-1)d]}
S
=
[
a
+
(
n
−
1
)
d
]
+
.
.
.
+
(
n
−
1
)
d
]
+
a
{\displaystyle S=[a+(n-1)d]+...+(n-1)d]+a}
2
S
=
[
2
a
+
(
n
−
1
)
d
]
n
{\displaystyle 2S=[2a+(n-1)d]n}
S
=
[
2
a
+
(
n
−
1
)
d
]
n
2
{\displaystyle S=[2a+(n-1)d]{\frac {n}{2}}}
Thí dụ
Dải số cấp số cộng có dạng tổng quát
1
,
2
,
3
,
.
.
.9
{\displaystyle 1,2,3,...9}
Tổng số của dải số
1
+
2
+
3
+
4
+
5
+
.
.
.9
=
50
{\displaystyle 1+2+3+4+5+...9=50}
Cách giải
S
=
(
1
+
9
)
+
(
2
+
8
)
+
(
3
+
7
)
+
(
4
+
6
)
+
(
5
+
5
)
=
10
(
5
)
=
50
{\displaystyle S=(1+9)+(2+8)+(3+7)+(4+6)+(5+5)=10(5)=50}
Tổng chuổi số cấp số nhân [ sửa ] Dạng tổng quát
a
+
a
r
+
a
r
2
+
a
r
3
+
a
r
4
+
…
+
a
r
n
=
∑
k
=
0
∞
(
a
r
k
)
{\displaystyle a+ar+ar^{2}+ar^{3}+ar^{4}+\ldots +ar^{n}=\sum _{k=0}^{\infty }(ar^{k})}
Chứng minh
∑
k
=
0
∞
(
a
r
k
)
=
a
+
a
r
+
a
r
2
+
a
r
3
+
a
r
4
+
…
+
a
r
n
=
a
(
1
−
r
n
)
1
−
r
{\displaystyle \sum _{k=0}^{\infty }(ar^{k})=a+ar+ar^{2}+ar^{3}+ar^{4}+\ldots +ar^{n}={\frac {a(1-r^{n})}{1-r}}}
S
=
a
+
a
r
+
a
r
2
+
a
r
3
+
.
.
.
+
a
r
n
−
1
{\displaystyle S=a+ar+ar^{2}+ar^{3}+...+ar^{n-1}}
r
S
=
a
r
+
a
r
2
+
a
r
3
+
a
r
4
+
.
.
.
+
a
r
n
{\displaystyle rS=ar+ar^{2}+ar^{3}+ar^{4}+...+ar^{n}}
S
−
r
S
=
a
−
a
r
n
{\displaystyle S-rS=a-ar^{n}}
S
=
a
(
1
−
r
n
)
1
−
r
{\displaystyle S={\frac {a(1-r^{n})}{1-r}}}
S
=
a
1
−
r
{\displaystyle S={\frac {a}{1-r}}}
n
<
1
{\displaystyle n<1}
Thí dụ
1
+
1.1
+
1.1
2
+
1.1
3
=
4
{\displaystyle 1+1.1+1.1^{2}+1.1^{3}=4}
1
+
1.2
+
1.2
2
+
1.2
3
=
1
+
2
+
4
+
8
=
15
{\displaystyle 1+1.2+1.2^{2}+1.2^{3}=1+2+4+8=15}
Tổng chuổi số Pascal [ sửa ] Công thức tổng quát lũy thừa n của một tổng
(
x
+
y
)
n
=
∑
r
=
0
n
(
n
r
)
x
r
y
n
−
r
{\displaystyle (x+y)^{n}=\sum _{r=0}^{n}{n \choose r}x^{r}y^{n-r}}
(
x
+
y
)
n
=
(
n
0
)
x
0
y
n
+
(
n
1
)
x
1
y
n
−
1
+
(
n
2
)
x
2
y
n
−
2
+
⋯
+
(
n
n
−
2
)
x
n
−
2
y
2
+
(
n
n
−
1
)
x
n
−
1
y
1
+
(
n
n
)
x
n
y
0
{\displaystyle (x+y)^{n}={n \choose 0}x^{0}y^{n}+{n \choose 1}x^{1}y^{n-1}+{n \choose 2}x^{2}y^{n-2}+\dots +{n \choose {n-2}}x^{n-2}y^{2}+{n \choose {n-1}}x^{n-1}y^{1}+{n \choose n}x^{n}y^{0}}
(
x
+
y
)
n
=
y
n
+
n
x
y
n
−
1
+
(
n
2
)
x
2
y
n
−
2
+
⋯
+
(
n
n
−
2
)
x
n
−
2
y
2
+
n
x
n
−
1
y
+
x
n
{\displaystyle (x+y)^{n}=y^{n}+nxy^{n-1}+{n \choose 2}x^{2}y^{n-2}+\dots +{n \choose {n-2}}x^{n-2}y^{2}+nx^{n-1}y+x^{n}}
Với
(
n
r
)
=
n
!
r
!
(
n
−
r
)
!
{\displaystyle {n \choose r}={\frac {n!}{r!(n-r)!}}}
Thí dụ
(
x
+
1
)
1
=
{\displaystyle (x+1)^{1}=}
1
x
+
1
{\displaystyle 1x+1}
(
x
+
1
)
2
=
{\displaystyle (x+1)^{2}=}
1
x
2
+
2
x
+
1
{\displaystyle 1x^{2}+2x+1}
(
x
+
1
)
3
=
{\displaystyle (x+1)^{3}=}
1
x
3
+
3
x
2
+
3
x
+
1
{\displaystyle 1x^{3}+3x^{2}+3x+1}
(
x
+
1
)
4
=
{\displaystyle (x+1)^{4}=}
1
x
4
+
4
x
3
+
6
x
2
+
4
x
+
1
{\displaystyle 1x^{4}+4x^{3}+6x^{2}+4x+1}
(
x
+
1
)
5
=
{\displaystyle (x+1)^{5}=}
1
x
5
+
5
x
4
+
10
x
3
+
10
x
2
+
5
x
+
1
{\displaystyle 1x^{5}+5x^{4}+10x^{3}+10x^{2}+5x+1}
1 1
1 2 1
1 3 3 1
1 4 6 4 1
1 5 10 10 5 1
1 6 15 20 15 6 1
1 7 21 35 35 21 7 1
1 8 28 56 70 56 28 8 1
1 9 36 84 126 126 84 36 9 1
1 10 45 120 210 252 210 120 45 10 1
1 11 55 165 330 462 462 330 165 55 11 1
Tổng chuổi số Taylor [ sửa ] Dạng tổng quát
f
(
a
)
+
f
′
(
a
)
1
!
(
x
−
a
)
+
f
″
(
a
)
2
!
(
x
−
a
)
2
+
f
‴
(
a
)
3
!
(
x
−
a
)
3
+
⋯
=
∑
n
=
0
∞
f
(
n
)
(
a
)
n
!
(
x
−
a
)
n
{\displaystyle f(a)+{\frac {f'(a)}{1!}}(x-a)+{\frac {f''(a)}{2!}}(x-a)^{2}+{\frac {f'''(a)}{3!}}(x-a)^{3}+\cdots =\sum _{n=0}^{\infty }{\frac {f^{(n)}(a)}{n!}}(x-a)^{n}}
Tổng dải số Fourier [ sửa ] Tổng chuổi số Fourier đại diện cho tổng chuổi số hàm số sóng sine
s
N
(
x
)
=
A
0
2
+
∑
n
=
1
N
A
n
⋅
sin
(
2
π
n
x
P
+
ϕ
n
)
,
for integer
N
≥
1.
{\displaystyle s_{N}(x)={\frac {A_{0}}{2}}+\sum _{n=1}^{N}A_{n}\cdot \sin \left({\tfrac {2\pi nx}{P}}+\phi _{n}\right),\quad {\text{for integer}}\ N\ \geq \ 1.}
Công thức tổng dải số [ sửa ]
∑
k
=
0
n
c
=
n
c
{\displaystyle \sum _{k=0}^{n}{c}=nc}
c
{\displaystyle c}
∑
k
=
0
n
k
=
n
(
n
+
1
)
2
{\displaystyle \sum _{k=0}^{n}{k}={\frac {n(n+1)}{2}}}
∑
k
=
0
n
k
2
=
n
(
n
+
1
)
(
2
n
+
1
)
6
{\displaystyle \sum _{k=0}^{n}{k^{2}}={\frac {n(n+1)(2n+1)}{6}}}
∑
k
=
0
n
k
3
=
n
2
(
n
+
1
)
2
4
{\displaystyle \sum _{k=0}^{n}{k^{3}}={\frac {n^{2}(n+1)^{2}}{4}}}
∑
n
=
0
∞
x
n
n
!
=
1
+
x
+
x
2
2
!
+
x
3
3
!
+
x
4
4
!
+
⋯
=
e
x
{\displaystyle \sum _{n=0}^{\infty }{\frac {x^{n}}{n!}}=1+x+{\frac {x^{2}}{2!}}+{\frac {x^{3}}{3!}}+{\frac {x^{4}}{4!}}+\cdots =e^{x}}
∑
n
=
1
∞
(
−
1
)
n
+
1
n
x
n
=
x
−
x
2
2
+
x
3
3
−
x
4
4
+
⋯
=
ln
(
1
+
x
)
for
|
x
|
<
1
{\displaystyle \sum _{n=1}^{\infty }{\frac {(-1)^{n+1}}{n}}x^{n}=x-{\frac {x^{2}}{2}}+{\frac {x^{3}}{3}}-{\frac {x^{4}}{4}}+\cdots =\ln(1+x)\quad {\text{ for }}|x|<1}
∑
n
=
0
∞
(
−
1
)
n
(
2
n
)
!
x
2
n
=
1
−
x
2
2
!
+
x
4
4
!
−
⋯
=
cos
(
x
)
for all
x
∈
C
{\displaystyle \sum _{n=0}^{\infty }{\frac {(-1)^{n}}{(2n)!}}x^{2n}=1-{\frac {x^{2}}{2!}}+{\frac {x^{4}}{4!}}-\cdots =\cos(x)\quad {\text{ for all }}x\in \mathbb {C} }
Biểu thức đại số [ sửa ]
Biểu thức Đơn thức Đa thức Đẳng thức Bất đẳng thức
2
x
{\displaystyle 2x}
5
x
y
z
{\displaystyle 5xyz}
2
x
+
5
y
{\displaystyle 2x+5y}
5
x
y
−
2
y
{\displaystyle 5xy-2y}
2
x
=
5
y
{\displaystyle 2x=5y}
5
x
y
=
2
y
{\displaystyle 5xy=2y}
2
x
{\displaystyle 2x}
5
y
{\displaystyle 5y}
5
x
y
{\displaystyle 5xy}
2
y
{\displaystyle 2y}
Hằng đẳng thức [ sửa ]
Hằng đẳng thức Công thức
Bình phương tổng 2 số đại số
(
a
+
b
)
2
=
(
a
+
b
)
(
a
+
b
)
=
a
2
+
a
b
+
a
b
+
b
2
=
a
2
+
2
a
b
+
b
2
{\displaystyle (a+b)^{2}=(a+b)(a+b)=a^{2}+ab+ab+b^{2}=a^{2}+2ab+b^{2}}
Bình phương hiệu 2 số đại số
(
a
−
b
)
2
=
(
a
−
b
)
(
a
−
b
)
=
a
2
−
a
b
−
a
b
+
b
2
=
a
2
−
2
a
b
+
b
2
{\displaystyle (a-b)^{2}=(a-b)(a-b)=a^{2}-ab-ab+b^{2}=a^{2}-2ab+b^{2}}
Tổng 2 bình phương
a
2
+
b
2
=
(
a
+
b
)
2
−
2
a
b
{\displaystyle a^{2}+b^{2}=(a+b)^{2}-2ab}
a
2
+
b
2
=
(
a
−
b
)
2
+
2
a
b
{\displaystyle a^{2}+b^{2}=(a-b)^{2}+2ab}
Hiệu 2 bình phương
a
2
−
b
2
=
(
a
+
b
)
(
a
−
b
)
{\displaystyle a^{2}-b^{2}=(a+b)(a-b)}
Tổng 2 lập phương
a
3
+
b
3
=
(
a
+
b
)
(
a
2
−
a
b
+
b
2
)
{\displaystyle a^{3}+b^{3}=(a+b)(a^{2}-ab+b^{2})}
Hiệu 2 lập phương
a
3
−
b
3
=
(
a
−
b
)
(
a
2
+
a
b
+
b
2
)
{\displaystyle a^{3}-b^{3}=(a-b)(a^{2}+ab+b^{2})}
Bất đẳng thức [ sửa ] Hàm số đại số [ sửa ] Tính chất [ sửa ]
Hàm số Công thức
Hàm số có dạng tổng quát
f
(
x
,
y
,
z
,
.
.
.
)
{\displaystyle f(x,y,z,...)}
Giá trị hàm số
f
(
x
,
y
,
z
,
.
.
.
)
=
C
{\displaystyle f(x,y,z,...)=C}
Loại hàm số [ sửa ]
Dạng hàm số Công thức Thí dụ
Hàm số tuần hoàn Periodic function
f
(
x
)
=
f
(
x
+
T
)
{\displaystyle f(x)=f(x+T)}
s
i
n
x
=
s
i
n
(
x
+
k
2
π
)
{\displaystyle sinx=sin(x+k2\pi )}
Hàm số chẳn even function
f
(
x
)
=
f
(
−
x
)
{\displaystyle f(x)=f(-x)}
y
(
x
)
=
|
x
|
{\displaystyle y(x)=|x|}
Hàm số lẽ odd function
f
(
x
)
=
−
f
(
x
)
{\displaystyle f(x)=-f(x)}
y
(
x
)
=
−
y
(
x
)
{\displaystyle y(x)=-y(x)}
Hàm số nghịch đảo inverse function
f
−
1
(
x
)
=
1
f
(
x
)
{\displaystyle f^{-1}(x)={\frac {1}{f(x)}}}
s
i
n
−
1
x
=
1
s
i
n
x
{\displaystyle sin^{-1}{x}={\frac {1}{sinx}}}
Hàm số trong hàm số composite function
f
(
x
)
=
f
(
g
(
x
)
)
{\displaystyle f(x)=f(g(x))}
Hàm số nhiều biến số parametric function
z
=
f
(
x
,
y
)
{\displaystyle z=f(x,y)}
Hàm số tương quan/]] recursive function
Phép toán hàm số [ sửa ] Đồ thị hàm số [ sửa ] Với mọi giá trị của x sẻ có một giá trị hàm số của x tương đương . Thí dụ, với hàm số f(x)=x ta có thể thiết lập bảng giá trị tương quan của x và hàm số của x . Khi đặt các giá trị của x và của f(x) trên đồ thị XY ta có thể vẻ được hình đường thẳng có độ góc bằng 1 đi qua điểm gốc ở tọa độ (0,0)
x -2 -1 0 1 2
Hình
F(x)=x -2 -1 0 1 2
Đồ thị hàm số Hình
Thẳng
Cong
Tròn
Lũy thừa
Log
Sin
Cos
Sec
Csc
Tan
Cot
Công thức toán [ sửa ]
Danh sách các hàm số Công thức
Hàm số đường thẳng
Y
=
Z
X
{\displaystyle Y=ZX}
y
−
y
o
=
Z
(
x
−
x
o
)
{\displaystyle y-y_{o}=Z(x-x_{o})}
y
=
y
o
+
Z
(
x
−
x
o
)
{\displaystyle y=y_{o}+Z(x-x_{o})}
Z
2
=
X
2
+
Y
2
{\displaystyle Z^{2}=X^{2}+Y^{2}}
Z
Z
2
=
X
Z
2
+
Y
Z
2
{\displaystyle {\frac {Z}{Z}}^{2}={\frac {X}{Z}}^{2}+{\frac {Y}{Z}}^{2}}
1
=
c
o
s
2
+
s
i
n
2
{\displaystyle 1=cos^{2}+sin^{2}}
1
=
s
e
c
2
+
t
a
n
2
{\displaystyle 1=sec^{2}+tan^{2}}
1
=
c
s
c
2
+
c
o
t
2
{\displaystyle 1=csc^{2}+cot^{2}}
c
o
s
θ
=
X
Z
{\displaystyle cos\theta ={\frac {X}{Z}}}
s
i
n
θ
=
Y
Z
{\displaystyle sin\theta ={\frac {Y}{Z}}}
s
e
c
θ
=
1
X
{\displaystyle sec\theta ={\frac {1}{X}}}
c
s
c
θ
=
1
Y
{\displaystyle csc\theta ={\frac {1}{Y}}}
t
a
n
θ
=
Y
X
{\displaystyle tan\theta ={\frac {Y}{X}}}
c
o
t
θ
=
X
Y
{\displaystyle cot\theta ={\frac {X}{Y}}}
y
=
a
x
n
{\displaystyle y=ax^{n}}
y
(
x
)
=
L
o
g
x
{\displaystyle y(x)=Logx}
y
(
x
)
=
a
x
n
+
a
n
−
1
x
n
−
1
+
.
.
.
+
a
0
x
0
{\displaystyle y(x)=ax^{n}+a_{n-1}x^{n-1}+...+a_{0}x^{0}}
Q
(
x
)
=
N
(
x
)
M
(
x
)
−
R
(
x
)
{\displaystyle Q(x)={\frac {N(x)}{M(x)}}-R(x)}
Biểu diển hàm số bằng tổng dải số Maclaurin [ sửa ] Maclaurin cho rằng mọi hàm số đều có thể biểu diển bằng tổng của dải số lũy thừa như sau
f
(
x
)
=
a
0
+
a
1
x
+
a
2
x
3
+
a
4
x
4
+
.
.
.
=
f
(
0
)
+
f
′
x
(
0
)
+
f
″
(
0
)
2
!
+
f
‴
(
0
)
3
!
+
.
.
.
{\displaystyle f(x)=a_{0}+a_{1}x+a_{2}x^{3}+a_{4}x^{4}+...=f(0)+f^{'}x(0)+{\frac {f^{''}(0)}{2!}}+{\frac {f^{'''}(0)}{3!}}+...}
Chứng minh
Khi x=0
f
(
0
)
=
a
0
{\displaystyle f(0)=a_{0}}
f
′
(
x
)
=
a
1
+
2
a
2
x
+
3
a
3
x
2
+
4
a
4
x
3
{\displaystyle f^{'}(x)=a_{1}+2a_{2}x+3a_{3}x^{2}+4a_{4}x^{3}}
f
′
(
0
)
=
a
1
{\displaystyle f^{'}(0)=a_{1}}
f
″
(
x
)
=
2
a
2
+
(
3
)
(
2
)
a
3
x
+
(
4
)
(
3
)
a
4
x
2
+
(
5
)
(
4
)
a
5
x
3
{\displaystyle f^{''}(x)=2a_{2}+(3)(2)a_{3}x+(4)(3)a_{4}x^{2}+(5)(4)a_{5}x^{3}}
f
″
(
0
)
=
2
a
2
{\displaystyle f^{''}(0)=2a_{2}}
a
2
=
f
″
(
0
)
2
{\displaystyle a_{2}={\frac {f^{''}(0)}{2}}}
f
‴
(
x
)
=
(
3
)
(
2
)
a
3
x
+
(
4
)
(
3
)
(
2
)
a
4
x
+
(
5
)
(
4
)
(
3
)
a
5
x
2
{\displaystyle f^{'''}(x)=(3)(2)a_{3}x+(4)(3)(2)a_{4}x+(5)(4)(3)a_{5}x^{2}}
f
‴
(
0
)
=
(
3
)
(
2
)
a
3
{\displaystyle f^{'''}(0)=(3)(2)a_{3}}
a
3
=
f
‴
(
0
)
3
!
{\displaystyle a_{3}={\frac {f^{'''}(0)}{3!}}}
a
0
,
a
−
1
,
a
−
2
{\displaystyle a_{0},a-1,a-2}
f
(
x
)
=
a
0
+
a
1
x
+
a
2
x
3
+
a
4
x
4
{\displaystyle f(x)=a_{0}+a_{1}x+a_{2}x^{3}+a_{4}x^{4}}
f
(
x
)
=
f
(
0
)
+
f
′
x
(
0
)
+
f
″
(
0
)
2
!
+
f
‴
(
0
)
3
!
{\displaystyle f(x)=f(0)+f^{'}x(0)+{\frac {f^{''}(0)}{2!}}+{\frac {f^{'''}(0)}{3!}}}
Toán giải tích - Phép toán hàm số [ sửa ] Với đường thẳng nghiêng đi qua 2 điểm (xo,yo) - (x,y) dưới đây
Ta có thể tính các loại toán sau
Biến đổi hàm số Biến đổi hàm số cho biết tỉ lệ thay đổi biến số y trên thay đổi biến số x có thể biểu diển bằng công thức toán dưới đây
a
=
Δ
y
Δ
x
=
y
−
y
o
x
−
x
o
{\displaystyle a={\frac {\Delta y}{\Delta x}}={\frac {y-y_{o}}{x-x_{o}}}}
a
=
Δ
f
(
x
)
Δ
x
=
f
(
x
+
Δ
x
)
−
f
(
x
)
(
x
+
Δ
x
)
−
x
{\displaystyle a={\frac {\Delta f(x)}{\Delta x}}={\frac {f(x+\Delta x)-f(x)}{(x+\Delta x)-x}}}
Với
Δ
x
=
x
−
x
o
=
(
x
+
Δ
x
)
−
x
{\displaystyle \Delta x=x-x_{o}=(x+\Delta x)-x}
Δ
y
=
y
−
y
o
=
Δ
f
(
x
)
=
f
(
x
+
Δ
x
)
−
f
(
x
)
{\displaystyle \Delta y=y-y_{o}=\Delta f(x)=f(x+\Delta x)-f(x)}
Diện tích dưới hình
s
=
Δ
x
y
+
Δ
x
Δ
y
2
=
Δ
x
[
y
+
Δ
y
2
]
=
Δ
x
[
f
(
x
)
+
Δ
f
(
x
)
2
]
{\displaystyle s=\Delta xy+\Delta x{\frac {\Delta y}{2}}=\Delta x[y+{\frac {\Delta y}{2}}]=\Delta x[f(x)+{\frac {\Delta f(x)}{2}}]}
s
=
Δ
x
[
f
(
x
)
+
Δ
f
(
x
)
2
]
=
Δ
x
2
[
2
f
(
x
)
+
f
(
x
+
Δ
x
)
−
f
(
x
)
]
=
Δ
x
2
[
f
(
x
)
+
f
(
x
+
Δ
x
)
]
{\displaystyle s=\Delta x[f(x)+{\frac {\Delta f(x)}{2}}]={\frac {\Delta x}{2}}[2f(x)+f(x+\Delta x)-f(x)]={\frac {\Delta x}{2}}[f(x)+f(x+\Delta x)]}
Với mọi đường cong bên dưới
Ta có thể tính các loại toán sau
Đạo hàm hàm số đường cong
d
d
x
f
(
x
)
=
f
′
(
x
)
=
lim
Δ
x
→
0
∑
Δ
f
(
x
)
Δ
x
=
lim
Δ
x
→
0
∑
f
(
x
+
Δ
x
)
−
f
(
x
)
Δ
x
{\displaystyle {\frac {d}{dx}}f(x)=f^{'}(x)=\lim _{\Delta x\to 0}\sum {\frac {\Delta f(x)}{\Delta x}}=\lim _{\Delta x\to 0}\sum {\frac {f(x+\Delta x)-f(x)}{\Delta x}}}
Tích phân xác định đường cong
∫
a
b
f
(
x
)
d
x
=
F
(
b
)
−
F
(
a
)
{\displaystyle \int _{a}^{b}f(x)\,dx=F(b)-F(a)}
Tích phân bất định đường cong
∫
f
(
x
)
d
x
=
lim
Δ
x
→
0
∑
(
f
(
x
)
+
Δ
f
(
x
)
2
)
Δ
x
=
F
(
x
)
+
C
{\displaystyle \int f(x)dx=\lim _{\Delta x\rightarrow 0}\sum (f(x)+{\frac {\Delta f(x)}{2}})\Delta x=F(x)+C}
Phương trình đại số [ sửa ] Dạng tổng quát [ sửa ] Phương trình có dạng tổng quát
f
(
x
,
y
,
z
,
.
.
.
)
=
0
{\displaystyle f(x,y,z,...)=0}
Giải phương trình [ sửa ] Quá trình tìm giá trị nghiệm số thỏa mản
Giải phương trình lũy thừa [ sửa ] Giải phương trình đạo hàm [ sửa ] Giải hệ phương trình tuyến tính [ sửa ] Dạng tổng quát của 2 biến số
a
11
x
+
a
12
y
=
a
1
n
{\displaystyle a_{11}x+a_{12}y=a_{1n}}
a
21
x
+
a
22
y
=
a
2
n
{\displaystyle a_{21}x+a_{22y}=a_{2n}}
Hình học Eucleur [ sửa ]
Góc Định nghỉa Ký hiệu Đơn vị Thí dụ
. Một chấm
A __ B
Đường thẳng [ sửa ]
Góc Định nghỉa Ký hiệu Đơn vị Thí dụ
Đường nối liền giửa 2 điểm tạo từ nhiều đoạn thẳng
AB
Góc Định nghỉa Ký hiệu Đơn vị Thí dụ
Khi hai đường thẳng cắt nhau tại một điểm
∠
{\displaystyle \angle }
1
r
a
d
=
180
o
π
{\displaystyle 1rad={\frac {180^{o}}{\pi }}}
1
o
=
π
180
o
{\displaystyle 1^{o}={\frac {\pi }{180^{o}}}}
∠
A
=
30
0
=
π
6
r
a
d
{\displaystyle \angle A=30^{0}={\frac {\pi }{6}}rad}
Đường thẳng [ sửa ] Định nghỉa [ sửa ] Tọa độ điểm đại số [ sửa ] Đường thẳng là một đường nối liền giửa 2 điểm (xo,yo) - (x,y)
Có độ dóc tính bằng
a
=
y
−
y
o
x
−
x
o
{\displaystyle a={\frac {y-y_{o}}{x-x_{o}}}}
Có thể biểu diển bằng hàm số toán đại số dưới đây
y
=
y
o
+
a
(
x
−
x
o
)
=
a
x
+
b
{\displaystyle y=y_{o}+a(x-x_{o})=ax+b}
Dạng đường thẳng [ sửa ] Đường thẳng vuông góc [ sửa ] Khi hai đường thẳng cắt nhau tại một điểm tạo nên một góc vuông 90o sẻ tạo ra hai Đường thẳng vuông góc voi nhau
A
B
⊥
C
D
{\displaystyle AB\perp CD}
⊥
{\displaystyle \perp }
Góc B đỏ = Góc B xanh = 90o o + 90o = 180o o - Góc B xanho - Góc B đỏ Đường thẳng song song [ sửa ] Hai đường thẳng không cắt nhau tại bất kỳ điểm nào là đường thẳng song song . Hai đường thẳng song song là hai đường thẳng không có điểm chung . Hai đường thẳng phân biệt thì hoặc cắt nhau hoặc song song . Ký hiệu đường thẳng song song
/
/
{\displaystyle //}
AB // CD Tính chất góc trong 2 đương thẳng song song
Nếu một đường thẳng cắt hai đường thẳng song song thì:
Hai góc so le trong bằng nhau;
Hai góc đồng vị bằng nhau;
Hai góc trong cùng phía bù nhau. Vector đường thẳng [ sửa ] Vector đường thẳng là một đường thẳng có hướng và có một độ dài . Vectơ đường thẳng có ký hiệu Vector → . Thí dụ, Vector
A
B
→
{\displaystyle {\vec {AB}}}
Mọi vector A đều có thể biểu diển bằng cường độ vector nhân với vector đơn vị như dưới đây
A
→
=
A
a
→
{\displaystyle {\vec {A}}=A{\vec {a}}}
Với
A
→
{\displaystyle {\vec {A}}}
A
→
=
A
{\displaystyle {\vec {A}}=A}
A
→
=
a
→
{\displaystyle {\vec {A}}={\vec {a}}}
A
=
A
→
a
{\displaystyle A={\frac {\vec {A}}{a}}}
Vector 1 đơn vị
a
→
=
A
→
a
{\displaystyle {\vec {a}}={\frac {\vec {A}}{a}}}
Thí dụ
Tam giác vuông Pythagore
Vector đương thẳng Công tức toán
Vector đương thẳng ngang
X
→
=
X
i
→
{\displaystyle {\vec {X}}=X{\vec {i}}}
Vector đương thẳng dọc
Y
→
=
Y
j
→
{\displaystyle {\vec {Y}}=Y{\vec {j}}}
Vector đương thẳng nghiêng
Z
→
=
Z
k
→
=
X
→
+
Y
→
=
X
i
→
+
Y
j
→
{\displaystyle {\vec {Z}}=Z{\vec {k}}={\vec {X}}+{\vec {Y}}=X{\vec {i}}+Y{\vec {j}}}
Vòng tròn Eucleur
Vector bán kín vòng tròn
R
→
=
R
r
→
=
Z
→
=
X
→
+
Y
→
=
X
i
→
+
Y
j
→
{\displaystyle {\vec {R}}=R{\vec {r}}={\vec {Z}}={\vec {X}}+{\vec {Y}}=X{\vec {i}}+Y{\vec {j}}}
Phép toán vector [ sửa ] Không gian 2 chiều [ sửa ] Cộng vector [ sửa ] Phép cộng hai vectơ : tổng của hai vectơ
A
B
→
{\displaystyle {\overrightarrow {AB}}}
C
D
→
{\displaystyle {\overrightarrow {CD}}}
di chuyển vectơ
C
D
→
{\displaystyle {\overrightarrow {CD}}}
C
D
→
{\displaystyle {\overrightarrow {CD}}}
A
B
→
{\displaystyle {\overrightarrow {AB}}}
C
≡
B
{\displaystyle C\equiv B}
A
D
→
{\displaystyle {\overrightarrow {AD}}}
di chuyển vectơ
C
D
→
{\displaystyle {\overrightarrow {CD}}}
A
B
→
{\displaystyle {\overrightarrow {AB}}}
A
B
→
{\displaystyle {\overrightarrow {AB}}}
C
D
→
{\displaystyle {\overrightarrow {CD}}}
Tính chất Vectơ Công thức
Tính chất giao hoán
a
→
+
b
→
=
b
→
+
a
→
{\displaystyle {\vec {a}}+{\vec {b}}={\vec {b}}+{\vec {a}}}
Tính chất kết hợp
(
a
→
+
b
→
)
+
c
→
=
a
→
+
(
b
→
+
c
→
)
{\displaystyle ({\vec {a}}+{\vec {b}})+{\vec {c}}={\vec {a}}+({\vec {b}}+{\vec {c}})}
Tính chất của vectơ-không
a
→
+
0
→
=
0
→
+
a
→
=
a
→
{\displaystyle {\vec {a}}+{\vec {0}}={\vec {0}}+{\vec {a}}={\vec {a}}}
Với 3 điểm A, B, C thẳng hàng ta có:
A
B
→
+
B
C
→
=
A
C
→
{\displaystyle {\vec {AB}}+{\vec {BC}}={\vec {AC}}}
I là trung điểm đoạn thẳng AB
⇔
A
I
→
+
B
I
→
=
0
→
{\displaystyle \Leftrightarrow {\vec {AI}}+{\vec {BI}}={\vec {0}}}
G là trọng tâm
△
A
B
C
{\displaystyle \vartriangle ABC}
⇔
G
A
→
+
G
B
→
+
G
C
→
=
0
→
{\displaystyle \Leftrightarrow {\vec {GA}}+{\vec {GB}}+{\vec {GC}}={\vec {0}}}
Trừ vector [ sửa ] Nhân vector [ sửa ] Với hai vectơ bất kì, với hằng số h và k, ta có
k
(
a
→
+
b
→
)
=
k
a
→
+
k
b
→
{\displaystyle k({\vec {a}}+{\vec {b}})=k{\vec {a}}+k{\vec {b}}}
(
h
+
k
)
a
→
=
h
a
→
+
k
a
→
{\displaystyle (h+k){\vec {a}}=h{\vec {a}}+k{\vec {a}}}
h
(
k
a
→
)
=
(
h
k
)
a
→
{\displaystyle h(k{\vec {a}})=(hk){\vec {a}}}
1.
a
→
=
a
→
{\displaystyle 1.{\vec {a}}={\vec {a}}}
(
−
1
)
.
a
→
=
−
a
→
{\displaystyle (-1).{\vec {a}}=-{\vec {a}}}
Không gian 3 chiều [ sửa ] Chấm 2 vector [ sửa ] Tích vô hướng của hai vectơ A = [A 1 , A 2 ,.. ., A n và B = [B 1 , B 2 ,.. ., B n được định nghĩa như sau
A
⋅
B
=
∑
i
=
1
n
A
i
B
i
=
A
1
B
1
+
A
2
B
2
+
⋯
+
A
n
B
n
{\displaystyle \mathbf {A} \cdot \mathbf {B} =\sum _{i=1}^{n}A_{i}B_{i}=A_{1}B_{1}+A_{2}B_{2}+\cdots +A_{n}B_{n}}
A
⋅
B
=
‖
A
‖
‖
B
‖
cos
(
θ
)
,
{\displaystyle \mathbf {A} \cdot \mathbf {B} =\|\mathbf {A} \|\ \|\mathbf {B} \|\cos(\theta ),}
A và B .Trường hợp đặc biệt,
Nếu A và B trực giao thì góc giữa chúng là 90°, do đó:
A
⋅
B
=
0.
{\displaystyle \mathbf {A} \cdot \mathbf {B} =0.}
Nếu chúng cùng hướng thì góc giữa chúng là 0°, do đó:
A
⋅
B
=
‖
A
‖
‖
B
‖
{\displaystyle \mathbf {A} \cdot \mathbf {B} =\left\|\mathbf {A} \right\|\,\left\|\mathbf {B} \right\|}
Suy ra tích vô hướng của vectơ A và chính nó là:
A
⋅
A
=
‖
A
‖
2
,
{\displaystyle \mathbf {A} \cdot \mathbf {A} =\left\|\mathbf {A} \right\|^{2},}
ta có:
‖
A
‖
=
A
⋅
A
,
{\displaystyle \left\|\mathbf {A} \right\|={\sqrt {\mathbf {A} \cdot \mathbf {A} }},}
là khoảng cách Euclid của vectơ, luôn có giá trị dương khi A khác 0 .
A = [A 1 , A 2 ,.. ., A n
‖
A
‖
=
∑
k
=
1
n
A
k
2
{\displaystyle \left\|\mathbf {A} \right\|={\sqrt {\sum _{k=1}^{n}A_{k}^{2}}}}
Cho a , b , và c là các vectơ và r là đại lượng vô hướng , tích vô hướng thỏa mãn các tính chất sau:.
Giao hoán :
a
⋅
b
=
b
⋅
a
,
{\displaystyle \mathbf {a} \cdot \mathbf {b} =\mathbf {b} \cdot \mathbf {a} ,}
được suy ra từ định nghĩa (θ góc giữa a và b ):
a
⋅
b
=
‖
a
‖
‖
b
‖
cos
θ
=
‖
b
‖
‖
a
‖
cos
θ
=
b
⋅
a
.
{\displaystyle \mathbf {a} \cdot \mathbf {b} =\left\|\mathbf {a} \right\|\left\|\mathbf {b} \right\|\cos \theta =\left\|\mathbf {b} \right\|\left\|\mathbf {a} \right\|\cos \theta =\mathbf {b} \cdot \mathbf {a} .}
Phân phối cho phép cộng vectơ:
a
⋅
(
b
+
c
)
=
a
⋅
b
+
a
⋅
c
.
{\displaystyle \mathbf {a} \cdot (\mathbf {b} +\mathbf {c} )=\mathbf {a} \cdot \mathbf {b} +\mathbf {a} \cdot \mathbf {c} .}
Dạng song tuyến
a
⋅
(
r
b
+
c
)
=
r
(
a
⋅
b
)
+
(
a
⋅
c
)
.
{\displaystyle \mathbf {a} \cdot (r\mathbf {b} +\mathbf {c} )=r(\mathbf {a} \cdot \mathbf {b} )+(\mathbf {a} \cdot \mathbf {c} ).}
Phép nhân vô hướng :
(
c
1
a
)
⋅
(
c
2
b
)
=
c
1
c
2
(
a
⋅
b
)
.
{\displaystyle (c_{1}\mathbf {a} )\cdot (c_{2}\mathbf {b} )=c_{1}c_{2}(\mathbf {a} \cdot \mathbf {b} ).}
Không có tính kết hợp bởi vì tích vô hướng giữa đại lượng vô hướng (a ⋅ b ) và vectơ (c ) không tồn tại, tức là biểu thức cho tính kết hợp: (a ⋅ b ) ⋅ c or a ⋅ (b ⋅ c ) là không hợp lệ.Trực giao :Hai vectơ khác vectơ không: a và b trực giao khi và chỉ khi a ⋅ b = 0
Hai vectơ trực giao trong không gian Euclid còn được gọi là vuông góc . Không có tính khử :
Tính khử cho phép nhân của các số được định nghĩa như sau: nếu
ab = ac b luôn luôn bằng c nếu a khác 0. Tích vô hướng không tuân theo tính khử:Nếu a ⋅ b = a ⋅ c a ≠ 0 a ⋅ (b − c ) = 0phân phối ; suy ra a trực giao với (b − c ) , tức là (b − c ) ≠ 0 b ≠ c Quy tắc đạo hàm tích: Nếu a và b là hàm số , thì đạo hàm ca ⋅ b a ′ ⋅ b + a ⋅ b ′
Tam giác có cạnh vectơ a and b , và góc giữa 2 vectơ là θ . Hai vectơ a và b có góc giữa hai vectơ là θ (như trong hình bên phải) tạo thành một tam giác có cạnh thứ ba là c = a − b c và chính nó là Định lý cos :
c
⋅
c
=
(
a
−
b
)
⋅
(
a
−
b
)
=
a
⋅
a
−
a
⋅
b
−
b
⋅
a
+
b
⋅
b
=
a
2
−
a
⋅
b
−
a
⋅
b
+
b
2
=
a
2
−
2
a
⋅
b
+
b
2
c
2
=
a
2
+
b
2
−
2
a
b
cos
θ
{\displaystyle {\begin{aligned}\mathbf {c} \cdot \mathbf {c} &=(\mathbf {a} -\mathbf {b} )\cdot (\mathbf {a} -\mathbf {b} )\\&=\mathbf {a} \cdot \mathbf {a} -\mathbf {a} \cdot \mathbf {b} -\mathbf {b} \cdot \mathbf {a} +\mathbf {b} \cdot \mathbf {b} \\&=a^{2}-\mathbf {a} \cdot \mathbf {b} -\mathbf {a} \cdot \mathbf {b} +b^{2}\\&=a^{2}-2\mathbf {a} \cdot \mathbf {b} +b^{2}\\c^{2}&=a^{2}+b^{2}-2ab\cos \theta \\\end{aligned}}}
Chéo 2 vector [ sửa ] Minh họa kết quả phép nhân vectơ trong hệ tọa độ bên phải
Xác định hướng của tích vectơ bằng Quy tắc bàn tay phải . Phép nhân vectơ của vectơ a và b được ký hiệu là a × b hay
[
a
→
,
b
→
]
{\displaystyle [{\vec {a}},{\vec {b}}]}
a
×
b
=
n
^
|
a
|
|
b
|
sin
θ
{\displaystyle \mathbf {a} \times \mathbf {b} =\mathbf {\hat {n}} \left|\mathbf {a} \right|\left|\mathbf {b} \right|\sin \theta }
với θ là góc giữa a và b (0° ≤ θ ≤ 180°) nằm trên mặt phẳng chứa a và b , và n là vectơ đơn vị vuông góc với a và b .
Thực tế có hai vectơ n thỏa mãn điều kiện vuông góc với a và b (khi a và b không cùng phương), vì nếu n vuông góc với a và b thì -n cũng vậy.
Việc chọn hướng của véctơ n phụ thuộc vào hệ tọa độ tuân theo quy tắc bàn tay trái hay quy tắc bàn tay phải . (a , b , a × b ) tuân cùng quy tắc với hệ tọa độ đang sử dụng để xác định các vectơ.
Vì kết quả phụ thuộc vào quy ước hệ tọa độ, nó được gọi là giả vectơ . May mắn là trong các hiện tượng tự nhiên, nhân vectơ luôn đi theo cặp đối chiều nhau, nên kết quả cuối cùng không phụ thuộc lựa chọn hệ tọa độ.
hệ trục tọa độ Oxyz, cho
n
1
→
=
(
A
1
,
B
1
,
C
1
)
{\displaystyle {\vec {n_{1}}}=(A_{1},B_{1},C_{1})}
n
2
→
=
(
A
2
,
B
2
,
C
2
)
{\displaystyle {\vec {n_{2}}}=(A_{2},B_{2},C_{2})}
[
n
1
→
,
n
2
→
]
=
(
|
B
1
C
1
B
2
C
2
|
,
|
C
1
A
1
C
2
A
2
|
,
|
A
1
B
1
A
2
B
2
|
)
{\displaystyle [{\vec {n_{1}}},{\vec {n_{2}}}]=({\begin{vmatrix}B_{1}&C_{1}\\B_{2}&C_{2}\end{vmatrix}},{\begin{vmatrix}C_{1}&A_{1}\\C_{2}&A_{2}\end{vmatrix}},{\begin{vmatrix}A_{1}&B_{1}\\A_{2}&B_{2}\end{vmatrix}})}
Ứng dụng [ sửa ] Nhiều công thức tính trong không gian vectơ ba chiều liên quan đến nhân vectơ, nhờ vào kết quả là vectơ vuông góc với hai vectơ đầu vào.
Diện tích hình bình hành ABCD:
S
=
|
[
A
B
→
;
A
D
→
]
|
=
A
B
.
A
D
.
s
i
n
(
A
)
{\displaystyle S=\left\vert [{\vec {AB}};{\vec {AD}}]\right\vert =AB.AD.sin(A)}
Thể tích khối hộp ABCDA'B'C'D':
V
=
|
[
A
B
→
;
A
D
→
]
⋅
A
A
′
→
|
{\displaystyle V=\left\vert [{\vec {AB}};{\vec {AD}}]\cdot {\vec {AA'}}\right\vert }
2 vector
u
→
{\displaystyle {\vec {u}}}
v
→
{\displaystyle {\vec {v}}}
⇔
{\displaystyle \Leftrightarrow }
[
u
→
;
v
→
]
=
0
→
{\displaystyle [{\vec {u}};{\vec {v}}]={\vec {0}}}
3 vector
u
→
{\displaystyle {\vec {u}}}
v
→
{\displaystyle {\vec {v}}}
w
→
{\displaystyle {\vec {w}}}
⇔
{\displaystyle \Leftrightarrow }
[
u
→
;
v
→
]
.
w
→
=
0
{\displaystyle [{\vec {u}};{\vec {v}}].{\vec {w}}=0}
Định nghỉa [ sửa ]
Góc Định nghỉa Ký hiệu Đơn vị Thí dụ
Khi hai đường thẳng cắt nhau tại một điểm
∠
{\displaystyle \angle }
1
r
a
d
=
180
o
π
{\displaystyle 1rad={\frac {180^{o}}{\pi }}}
1
o
=
π
180
o
{\displaystyle 1^{o}={\frac {\pi }{180^{o}}}}
∠
A
=
30
0
=
π
6
r
a
d
{\displaystyle \angle A=30^{0}={\frac {\pi }{6}}rad}
Thể loại góc [ sửa ]
Góc Hình Định nghỉa
Góc nhọn
Góc nhọn là góc nhỏ hơn 90°
Góc vuông
Góc vuông là góc bằng 90° (1/4 vòng tròn);
Góc tù
Góc tù là góc lớn hơn 90° nhưng nhỏ hơn 180°
Góc bẹt
Góc bẹt là góc 180° (1/2 vòng tròn).
Góc phản
Góc phản là góc lớn hơn 180° nhưng nhỏ hơn 360°
Góc đầy
Góc đầy là góc bằng 360° (toàn bộ vòng tròn).
Hình tam giác [ sửa ] Một tam giác với các thành phần trong định lý sin 3 điểm .
A
,
B
,
C
{\displaystyle A,B,C}
3 cạnh .
A
B
,
B
C
,
C
A
{\displaystyle AB,BC,CA}
3 góc .
∠
A
,
∠
B
,
∠
C
{\displaystyle \angle A,\angle B,\angle C}
Chu vi Diện tích Thể tích [ sửa ]
Chu vi
Diện tích
Thể tích
a
+
b
+
c
{\displaystyle a+b+c}
b
a
2
{\displaystyle {\frac {ba}{2}}}
a
b
h
2
{\displaystyle ab{\frac {h}{2}}}
Tam giác thường [ sửa ] Định lý Sin [ sửa ] Trong lượng giác , định lý sin (hay định luật sin , công thức sin ) là một phương trình biểu diễn mối quan hệ giữa chiều dài các cạnh của một tam giác bất kì với sin của các góc tương ứng. Định lý sin được biểu diễn dưới dạng
a
sin
A
=
b
sin
B
=
c
sin
C
{\displaystyle {\frac {a}{\sin A}}\,=\,{\frac {b}{\sin B}}\,=\,{\frac {c}{\sin C}}\!}
trong đó a , b , c là chiều dài các cạnh, và A , B , C là các góc đối diện (xem hình vẽ). Phương trình cũng có thể được viết dưới dạng nghịch đảo:
sin
A
a
=
sin
B
b
=
sin
C
c
.
{\displaystyle {\frac {\sin A}{a}}\,=\,{\frac {\sin B}{b}}\,=\,{\frac {\sin C}{c}}.\!}
Định lý Cosin [ sửa ]
a
2
=
b
2
+
c
2
−
2
b
c
cos
α
{\displaystyle a^{2}=b^{2}+c^{2}-2bc\cos \alpha \,}
b
2
=
a
2
+
c
2
−
2
a
c
cos
β
{\displaystyle b^{2}=a^{2}+c^{2}-2ac\cos \beta \,}
c
2
=
a
2
+
b
2
−
2
a
b
cos
γ
{\displaystyle c^{2}=a^{2}+b^{2}-2ab\cos \gamma \,}
Tam giác vuông [ sửa ] Tam giác vuông là một loại tam giác có 2 cạnh vuông góc với nhau cắt nhau tại một điểm tạo nên một góc vuông bằng
90
o
{\displaystyle 90^{o}}
c - Cạnh huyền
a - Cạnh đối
b - Cạnh kề
Tam giác có 2 cạnh vuông góc với nhau tạo ra một góc vuông 90o
∠
C
=
90
o
{\displaystyle \angle C=90^{o}}
A
C
¯
⊥
C
B
¯
{\displaystyle {\overline {AC}}\perp {\overline {CB}}}
Định lý tam giác vuông [ sửa ] Tam giác có 1 góc vuông là tam giác vuông
Tam giác có 2 góc nhọn phụ nhau là tam giác vuông
Tam giác có bình phương độ dài 1 cạnh bằng tổng bình phương độ dài 2 cạnh kia là tam giác vuông (định lý Pytago đảo)
Tam giác có đường trung tuyến ứng với 1 cạnh bằng nửa cạnh ấy là tam giác vuông
Tam giác nội tiếp đường tròn có 1 cạnh là đường kính thì tam giác đó vuông
Tam giác có cạnh đối diện góc 30° bằng một nửa một cạnh khác trong tam giác thì tam giác đó vuông. Định lý Pytago [ sửa ] Định lý Pytago phát biểu rằng:
Tổng diện tích của hai hình vuông vẽ trên cạnh kề của một tam giác vuông bằng diện tích hình vuông vẽ trên cạnh huyền của tam giác này . Nó được thể hiện bằng phương trình
a
2
+
b
2
=
c
2
{\displaystyle \displaystyle a^{2}+b^{2}=c^{2}}
Trong đó, c là chiều dài của cạnh huyền và a và b là chiều dài của hai cạnh còn lại.
Hàm số lượng giác [ sửa ] Tương quan các cạnh và góc
Hàm số góc lượng giác Tỉ lệ cạnh Đồ thị
Cosine
X
Z
=
cos
θ
{\displaystyle {\frac {X}{Z}}=\cos \theta }
Sine
Y
Z
=
sin
θ
{\displaystyle {\frac {Y}{Z}}=\sin \theta }
Cosine
1
X
=
sec
θ
{\displaystyle {\frac {1}{X}}=\sec \theta }
Cosecant
1
Y
=
csc
θ
{\displaystyle {\frac {1}{Y}}=\csc \theta }
Tangent
Y
X
=
tan
θ
{\displaystyle {\frac {Y}{X}}=\tan \theta }
Cotangent
X
Y
=
cot
θ
{\displaystyle {\frac {X}{Y}}=\cot \theta }
Tam giác vuông trên đồ thị XY [ sửa ]
Hàm số cạnh
Độ dài cạnh ngang
X
=
Y
Z
{\displaystyle X={\frac {Y}{Z}}}
x
−
x
o
{\displaystyle x-x_{o}}
Δ
x
{\displaystyle \Delta x}
Z
cos
θ
{\displaystyle Z\cos \theta }
Độ dài cạnh dọc
Y
=
Z
X
{\displaystyle Y=ZX}
y
−
y
o
{\displaystyle y-y_{o}}
Δ
y
{\displaystyle \Delta y}
Z
sin
θ
{\displaystyle Z\sin \theta }
Độ dóc
Z
=
Y
X
{\displaystyle Z={\frac {Y}{X}}}
y
−
y
o
x
−
x
o
{\displaystyle {\frac {y-y_{o}}{x-x_{o}}}}
Δ
y
Δ
x
{\displaystyle {\frac {\Delta y}{\Delta x}}}
T
a
n
θ
{\displaystyle Tan\theta }
Độ nghiêng
θ
=
tan
−
1
Z
{\displaystyle \theta =\tan ^{-1}Z}
θ
=
tan
−
1
Y
X
{\displaystyle \theta =\tan ^{-1}{\frac {Y}{X}}}
Vector đương thẳng ngang
X
→
=
x
i
→
{\displaystyle {\vec {X}}=x{\vec {i}}}
(
x
−
x
o
)
i
→
{\displaystyle (x-x_{o}){\vec {i}}}
Z
cos
θ
i
→
{\displaystyle Z\cos \theta {\vec {i}}}
Vector đương thẳng dọc
Y
→
=
y
i
→
{\displaystyle {\vec {Y}}=y{\vec {i}}}
(
y
−
y
o
)
i
→
{\displaystyle (y-y_{o}){\vec {i}}}
Z
sin
θ
i
→
{\displaystyle Z\sin \theta {\vec {i}}}
Vector đương thẳng nghiêng
Z
→
=
z
k
→
{\displaystyle {\vec {Z}}=z{\vec {k}}}
(
z
−
z
o
)
k
→
{\displaystyle (z-z_{o}){\vec {k}}}
Hàm số Đường thẳng nghiêng ở độ dóc Z
Y
=
Z
X
{\displaystyle Y=ZX}
y
=
y
o
+
Z
(
x
−
x
o
)
{\displaystyle y=y_{o}+Z(x-x_{o})}
Z
∠
θ
=
X
2
+
Y
2
∠
T
a
n
−
1
Y
X
{\displaystyle Z\angle \theta ={\sqrt {X^{2}+Y^{2}}}\angle Tan^{-1}{\frac {Y}{X}}}
s
=
X
(
y
o
+
Y
2
)
=
X
(
y
o
+
Z
X
2
)
=
X
(
y
−
Z
X
2
)
=
y
2
−
y
o
2
2
Z
{\displaystyle s=X(y_{o}+{\frac {Y}{2}})=X(y_{o}+{\frac {ZX}{2}})=X(y-{\frac {ZX}{2}})={\frac {y^{2}-y_{o}^{2}}{2Z}}}
Hình cong [ sửa ] Độ nghiêng đường thẳng
a
=
f
(
x
+
Δ
x
)
−
f
(
x
)
(
x
+
Δ
x
)
−
x
=
Δ
f
(
x
)
Δ
x
{\displaystyle a={\frac {f(x+\Delta x)-f(x)}{(x+\Delta x)-x}}={\frac {\Delta f(x)}{\Delta x}}}
Diện tích dưới hình
s
=
Δ
x
[
f
(
x
)
+
Δ
f
(
x
)
2
]
{\displaystyle s=\Delta x[f(x)+{\frac {\Delta f(x)}{2}}]}
Khi
Δ
x
−
>
0
{\displaystyle \Delta x->0}
Độ nghiêng đường thẳng
a
(
x
)
=
lim
Δ
x
→
0
∑
Δ
f
(
x
)
Δ
x
=
d
d
x
f
(
x
)
=
f
′
(
x
)
{\displaystyle a(x)=\lim _{\Delta x\to 0}\sum {\frac {\Delta f(x)}{\Delta x}}={\frac {d}{dx}}f(x)=f^{'}(x)}
Diện tích dưới hình
s
(
x
)
=
lim
Δ
x
→
0
∑
(
f
(
x
)
+
Δ
f
(
x
)
2
)
Δ
x
=
∫
f
(
x
)
d
x
=
F
(
x
)
+
C
{\displaystyle s(x)=\lim _{\Delta x\rightarrow 0}\sum (f(x)+{\frac {\Delta f(x)}{2}})\Delta x=\int f(x)dx=F(x)+C}
s
(
x
)
=
∫
a
b
f
(
x
)
d
x
=
F
(
b
)
−
F
(
a
)
{\displaystyle s(x)=\int \limits _{a}^{b}f(x)dx=F(b)-F(a)}
s
(
x
)
=
∫
a
b
f
(
x
)
d
x
=
∫
a
c
f
(
x
)
d
x
+
∫
c
b
f
(
x
)
d
x
{\displaystyle s(x)=\int \limits _{a}^{b}f(x)dx=\int \limits _{a}^{c}f(x)dx+\int \limits _{c}^{b}f(x)dx}
−
s
(
x
)
=
∫
b
a
f
(
x
)
d
x
=
F
(
a
)
−
F
(
b
)
{\displaystyle -s(x)=\int \limits _{b}^{a}f(x)dx=F(a)-F(b)}
Hàm số lượng giác cơ bản [ sửa ] Định nghỉa [ sửa ] 6 Công thức hàm số lượng giác cơ bản định nghỉa tương quan giửa các cạnh và góc trong tam giác vuông
Hàm số lượng giác cơ bản
cos
x
{\displaystyle \cos x}
sin
x
{\displaystyle \sin x}
tan
x
{\displaystyle \tan x}
cot
x
{\displaystyle \cot x}
sec
x
{\displaystyle \sec x}
csc
x
{\displaystyle \csc x}
b
c
{\displaystyle {\frac {b}{c}}}
a
c
{\displaystyle {\frac {a}{c}}}
a
b
{\displaystyle {\frac {a}{b}}}
b
a
{\displaystyle {\frac {b}{a}}}
1
b
{\displaystyle {\frac {1}{b}}}
1
a
{\displaystyle {\frac {1}{a}}}
Tính chất [ sửa ] Tuần hoàn [ sửa ]
sin
(
x
)
=
sin
(
x
+
2
k
π
)
{\displaystyle \sin(x)=\sin(x+2k\pi )\,}
sin
(
−
x
)
=
−
sin
(
x
)
{\displaystyle \sin(-x)=-\sin(x)\,}
sin
(
x
)
=
cos
(
π
2
−
x
)
{\displaystyle \sin(x)=\cos \left({\frac {\pi }{2}}-x\right)}
Đối xứng [ sửa ]
cos
(
x
)
=
cos
(
x
+
2
k
π
)
{\displaystyle \cos(x)=\cos(x+2k\pi )\,}
cos
(
−
x
)
=
cos
(
x
)
{\displaystyle \cos(-x)=\;\cos(x)\,}
cos
(
x
)
=
sin
(
π
2
−
x
)
{\displaystyle \cos(x)=\sin \left({\frac {\pi }{2}}-x\right)}
Tịnh tiến [ sửa ]
tan
(
x
)
=
tan
(
x
+
k
π
)
{\displaystyle \tan(x)=\tan(x+k\pi )\,}
tan
(
−
x
)
=
−
tan
(
x
)
{\displaystyle \tan(-x)=-\tan(x)\,}
tan
(
x
)
=
cot
(
π
2
−
x
)
{\displaystyle \tan(x)=\cot \left({\frac {\pi }{2}}-x\right)}
cot
(
−
x
)
=
−
cot
(
x
)
{\displaystyle \cot(-x)=-\cot(x)\,}
a
sin
x
+
b
cos
x
=
a
2
+
b
2
⋅
sin
(
x
+
φ
)
{\displaystyle a\sin x+b\cos x={\sqrt {a^{2}+b^{2}}}\cdot \sin(x+\varphi )}
với
φ
=
{
a
r
c
t
a
n
(
b
/
a
)
,
n
e
^
´
u
a
≥
0
;
π
+
a
r
c
t
a
n
(
b
/
a
)
,
n
e
^
´
u
a
<
0.
{\displaystyle \varphi =\left\{{\begin{matrix}{\rm {arctan}}(b/a),&&{\mbox{n}}{\acute {\hat {\mbox{e}}}}{\mbox{u}}\ a\geq 0;\;\\\pi +{\rm {arctan}}(b/a),&&{\mbox{n}}{\acute {\hat {\mbox{e}}}}{\mbox{u}}\ a<0.\;\end{matrix}}\right.\;}
Góc bội [ sửa ]
sin
(
2
x
)
=
2
sin
(
x
)
cos
(
x
)
{\displaystyle \sin(2x)=2\sin(x)\cos(x)\,}
cos
(
2
x
)
=
cos
2
(
x
)
−
sin
2
(
x
)
=
2
cos
2
(
x
)
−
1
=
1
−
2
sin
2
(
x
)
{\displaystyle \cos(2x)=\cos ^{2}(x)-\sin ^{2}(x)=2\cos ^{2}(x)-1=1-2\sin ^{2}(x)\,}
tan
(
2
x
)
=
2
tan
(
x
)
1
−
tan
2
(
x
)
{\displaystyle \tan(2x)={\frac {2\tan(x)}{1-\tan ^{2}(x)}}}
sin
(
3
x
)
=
3
sin
(
x
)
−
4
sin
3
(
x
)
{\displaystyle \sin(3x)=3\sin(x)-4\sin ^{3}(x)}
cos
(
3
x
)
=
4
cos
3
(
x
)
−
3
cos
(
x
)
{\displaystyle \cos(3x)=4\cos ^{3}(x)-3\cos(x)}
Nếu Tn là đa thức Chebyshev bậc n thì
cos
(
n
x
)
=
T
n
(
cos
(
x
)
)
.
{\displaystyle \cos(nx)=T_{n}(\cos(x)).\,}
công thức de Moivre:
cos
(
n
x
)
+
i
sin
(
n
x
)
=
(
cos
(
x
)
+
i
sin
(
x
)
)
n
{\displaystyle \cos(nx)+i\sin(nx)=(\cos(x)+i\sin(x))^{n}\,}
Hàm hạt nhân Dirichlet Dn(x) sẽ xuất hiện trong các công thức sau:
1
+
2
cos
(
x
)
+
2
cos
(
2
x
)
+
2
cos
(
3
x
)
+
⋯
+
2
cos
(
n
x
)
{\displaystyle 1+2\cos(x)+2\cos(2x)+2\cos(3x)+\cdots +2\cos(nx)\;}
=
sin
(
(
n
+
1
2
)
x
)
sin
(
x
/
2
)
{\displaystyle ={\frac {\sin \left(\left(n+{\frac {1}{2}}\right)x\right)}{\sin(x/2)}}\;}
Hay theo công thức hồi quy:
sin
(
n
x
)
=
2
sin
(
(
n
−
1
)
x
)
cos
(
x
)
−
sin
(
(
n
−
2
)
x
)
{\displaystyle \sin(nx)=2\sin((n-1)x)\cos(x)-\sin((n-2)x)}
cos
(
n
x
)
=
2
cos
(
(
n
−
1
)
x
)
cos
(
x
)
−
cos
(
(
n
−
2
)
x
)
{\displaystyle \cos(nx)=2\cos((n-1)x)\cos(x)-\cos((n-2)x)}
Góc chia đôi [ sửa ]
cos
(
x
2
)
=
±
1
+
cos
(
x
)
2
{\displaystyle \cos \left({\frac {x}{2}}\right)=\pm \,{\sqrt {\frac {1+\cos(x)}{2}}}}
sin
(
x
2
)
=
±
1
−
cos
(
x
)
2
{\displaystyle \sin \left({\frac {x}{2}}\right)=\pm \,{\sqrt {\frac {1-\cos(x)}{2}}}}
tan
(
x
2
)
=
sin
(
x
/
2
)
cos
(
x
/
2
)
=
±
1
−
cos
x
1
+
cos
x
.
{\displaystyle \tan \left({\frac {x}{2}}\right)={\sin(x/2) \over \cos(x/2)}=\pm \,{\sqrt {1-\cos x \over 1+\cos x}}.\qquad \qquad }
x , rồi dùng định lý Pytago để đơn giản hóa:
tan
(
x
2
)
=
±
(
1
−
cos
x
)
(
1
+
cos
x
)
(
1
+
cos
x
)
(
1
+
cos
x
)
=
±
1
−
cos
2
x
(
1
+
cos
x
)
2
{\displaystyle \tan \left({\frac {x}{2}}\right)=\pm \,{\sqrt {(1-\cos x)(1+\cos x) \over (1+\cos x)(1+\cos x)}}=\pm \,{\sqrt {1-\cos ^{2}x \over (1+\cos x)^{2}}}}
=
sin
x
1
+
cos
x
.
{\displaystyle ={\sin x \over 1+\cos x}.}
Tương tự, lại nhân với mẫu số và tử số của phương trình (1) bởi 1 − cos x , rồi đơn giản hóa:
tan
(
x
2
)
=
±
(
1
−
cos
x
)
(
1
−
cos
x
)
(
1
+
cos
x
)
(
1
−
cos
x
)
=
±
(
1
−
cos
x
)
2
(
1
−
cos
2
x
)
{\displaystyle \tan \left({\frac {x}{2}}\right)=\pm \,{\sqrt {(1-\cos x)(1-\cos x) \over (1+\cos x)(1-\cos x)}}=\pm \,{\sqrt {(1-\cos x)^{2} \over (1-\cos ^{2}x)}}}
=
1
−
cos
x
sin
x
.
{\displaystyle ={1-\cos x \over \sin x}.}
Suy ra:
tan
(
x
2
)
=
sin
(
x
)
1
+
cos
(
x
)
=
1
−
cos
(
x
)
sin
(
x
)
.
{\displaystyle \tan \left({\frac {x}{2}}\right)={\frac {\sin(x)}{1+\cos(x)}}={\frac {1-\cos(x)}{\sin(x)}}.}
Nếu
t
=
tan
(
x
2
)
,
{\displaystyle t=\tan \left({\frac {x}{2}}\right),}
thì:
sin
(
x
)
=
2
t
1
+
t
2
{\displaystyle \sin(x)={\frac {2t}{1+t^{2}}}}
and
cos
(
x
)
=
1
−
t
2
1
+
t
2
{\displaystyle \cos(x)={\frac {1-t^{2}}{1+t^{2}}}}
and
e
i
x
=
1
+
i
t
1
−
i
t
.
{\displaystyle e^{ix}={\frac {1+it}{1-it}}.}
Tổng 2 góc [ sửa ]
sin
(
x
+
y
)
=
sin
x
cos
y
+
cos
x
sin
y
{\displaystyle \sin \left(x+y\right)=\sin x\cos y+\cos x\sin y}
cos
(
x
+
y
)
=
cos
x
cos
y
−
sin
x
sin
y
{\displaystyle \cos \left(x+y\right)=\cos x\cos y-\sin x\sin y}
sin
x
+
sin
y
=
2
sin
(
x
+
y
2
)
cos
(
x
−
y
2
)
{\displaystyle \sin x+\sin y=2\sin \left({\frac {x+y}{2}}\right)\cos \left({\frac {x-y}{2}}\right)}
cos
x
+
cos
y
=
2
cos
(
x
+
y
2
)
cos
(
x
−
y
2
)
{\displaystyle \cos x+\cos y=2\cos \left({\frac {x+y}{2}}\right)\cos \left({\frac {x-y}{2}}\right)}
tan
x
+
tan
y
=
sin
(
x
+
y
)
cos
x
cos
y
{\displaystyle \tan x+\tan y={\frac {\sin \left(x+y\right)}{\cos x\cos y}}}
cot
x
+
cot
y
=
sin
(
x
+
y
)
sin
x
sin
y
{\displaystyle \cot x+\cot y={\frac {\sin \left(x+y\right)}{\sin x\sin y}}}
Hiệu 2 góc [ sửa ]
sin
(
x
−
y
)
=
sin
x
cos
y
−
cos
x
sin
y
{\displaystyle \sin \left(x-y\right)=\sin x\cos y-\cos x\sin y}
cos
(
x
−
y
)
=
cos
x
cos
y
+
sin
x
sin
y
{\displaystyle \cos \left(x-y\right)=\cos x\cos y+\sin x\sin y}
sin
x
−
sin
y
=
2
cos
(
x
+
y
2
)
sin
(
x
−
y
2
)
{\displaystyle \sin x-\sin y=2\cos \left({\frac {x+y}{2}}\right)\sin \left({\frac {x-y}{2}}\right)}
cos
x
−
cos
y
=
−
2
sin
(
x
+
y
2
)
sin
(
x
−
y
2
)
{\displaystyle \cos x-\cos y=-2\sin \left({\frac {x+y}{2}}\right)\sin \left({\frac {x-y}{2}}\right)}
tan
x
−
tan
y
=
sin
(
x
−
y
)
cos
x
cos
y
{\displaystyle \tan x-\tan y={\frac {\sin \left(x-y\right)}{\cos x\cos y}}}
cot
x
−
cot
y
=
−
sin
(
x
−
y
)
sin
x
sin
y
{\displaystyle \cot x-\cot y={\frac {-\sin \left(x-y\right)}{\sin x\sin y}}}
Tích 2 góc [ sửa ]
cos
(
x
)
cos
(
y
)
=
cos
(
x
+
y
)
+
cos
(
x
−
y
)
2
{\displaystyle \cos \left(x\right)\cos \left(y\right)={\cos \left(x+y\right)+\cos \left(x-y\right) \over 2}\;}
sin
(
x
)
sin
(
y
)
=
cos
(
x
−
y
)
−
cos
(
x
+
y
)
2
{\displaystyle \sin \left(x\right)\sin \left(y\right)={\cos \left(x-y\right)-\cos \left(x+y\right) \over 2}\;}
sin
(
x
)
cos
(
y
)
=
sin
(
x
−
y
)
+
sin
(
x
+
y
)
2
{\displaystyle \sin \left(x\right)\cos \left(y\right)={\sin \left(x-y\right)+\sin \left(x+y\right) \over 2}\;}
Lũy thừa góc [ sửa ]
cos
2
(
x
)
=
1
+
cos
(
2
x
)
2
{\displaystyle \cos ^{2}(x)={1+\cos(2x) \over 2}}
sin
2
(
x
)
=
1
−
cos
(
2
x
)
2
{\displaystyle \sin ^{2}(x)={1-\cos(2x) \over 2}}
sin
2
(
x
)
cos
2
2
(
x
)
=
1
−
cos
(
4
x
)
4
{\displaystyle \sin ^{2}(x)\cos ^{2}2(x)={1-\cos(4x) \over 4}}
sin
3
(
x
)
=
2
sin
2
(
x
)
−
sin
(
3
x
)
4
{\displaystyle \sin ^{3}(x)={\frac {2\sin 2(x)-\sin(3x)}{4}}}
cos
3
(
x
)
=
3
cos
(
x
)
+
cos
(
3
x
)
4
{\displaystyle \cos ^{3}(x)={\frac {3\cos(x)+\cos(3x)}{4}}}
Hàm số lượng giác nghịch [ sửa ] Hàm số lượng đường thẳng [ sửa ] Hàm số lượng đường thẳng nghiêng
Z
=
z
∠
θ
=
X
2
+
Y
2
∠
t
a
n
−
1
Y
X
{\displaystyle Z=z\angle \theta ={\sqrt {X^{2}+Y^{2}}}\angle tan^{-1}{\frac {Y}{X}}}
Hàm số lượng đường thẳng dọc
Y
=
y
∠
90
{\displaystyle Y=y\angle 90}
Hàm số lượng đường thẳng ngang
X
=
x
∠
0
{\displaystyle X=x\angle 0}
Hàm số lượng đường tròn [ sửa ] Hàm số lượng đường tròn Z đơn vị
R
=
θ
{\displaystyle R=\theta }
Z
2
=
X
2
+
Y
2
{\displaystyle Z^{2}=X^{2}+Y^{2}}
Hàm số lượng đường tròn 1 đơn vị
1
=
(
X
Z
)
2
+
(
Y
Z
)
2
=
c
o
s
2
x
+
s
i
n
2
x
=
s
e
c
2
x
−
t
a
n
2
x
=
c
s
c
2
x
−
c
o
t
2
x
{\displaystyle 1=({\frac {X}{Z}})^{2}+({\frac {Y}{Z}})^{2}=cos^{2}x+sin^{2}x=sec^{2}x-tan^{2}x=csc^{2}x-cot^{2}x}
Vector [ sửa ] Vector đại diện cho một đường thẳng có hướng và có một độ dài . Vectơ có ký hiệu → . Thí dụ, Vector
A
B
→
{\displaystyle {\vec {AB}}}
Tính chất [ sửa ] Mọi vector A đều có thể biểu diển bằng cường độ vector nhân với vector đơn vị như dưới đây
A
→
=
A
a
→
{\displaystyle {\vec {A}}=A{\vec {a}}}
Với
A
→
{\displaystyle {\vec {A}}}
A
→
=
A
{\displaystyle {\vec {A}}=A}
A
→
=
a
→
{\displaystyle {\vec {A}}={\vec {a}}}
A
=
A
→
a
{\displaystyle A={\frac {\vec {A}}{a}}}
Vector 1 đơn vị
a
→
=
A
→
a
{\displaystyle {\vec {a}}={\frac {\vec {A}}{a}}}
Thí dụ [ sửa ] Trong hệ tọa độ XY
Vector chuyển động thẳng hàng ngang
X
→
=
X
i
→
{\displaystyle {\vec {X}}=X{\vec {i}}}
Vector chuyển động thẳng hàng dọc
Y
→
=
Y
j
→
{\displaystyle {\vec {Y}}=Y{\vec {j}}}
Vector chuyển động thẳng hàng nghiêng
Z
→
=
Z
k
→
=
X
→
+
Y
→
=
X
i
→
+
Y
j
→
{\displaystyle {\vec {Z}}=Z{\vec {k}}={\vec {X}}+{\vec {Y}}=X{\vec {i}}+Y{\vec {j}}}
Vector chuyển động tròn
R
→
=
R
r
→
=
Z
→
=
X
→
+
Y
→
=
X
i
→
+
Y
j
→
{\displaystyle {\vec {R}}=R{\vec {r}}={\vec {Z}}={\vec {X}}+{\vec {Y}}=X{\vec {i}}+Y{\vec {j}}}
Chuyển Động s v a
Cong
s
(
t
)
{\displaystyle s(t)}
d
d
t
s
(
t
)
{\displaystyle {\frac {d}{dt}}s(t)}
d
2
d
t
2
s
(
t
)
{\displaystyle {\frac {d^{2}}{dt^{2}}}s(t)}
X
→
=
X
i
→
{\displaystyle {\vec {X}}=X{\vec {i}}}
d
d
t
X
→
=
d
X
d
t
i
→
=
v
x
i
→
{\displaystyle {\frac {d}{dt}}{\vec {X}}={\frac {dX}{dt}}{\vec {i}}=v_{x}{\vec {i}}}
d
2
d
t
2
X
→
=
d
2
X
d
t
2
i
→
=
a
x
i
→
{\displaystyle {\frac {d^{2}}{dt^{2}}}{\vec {X}}={\frac {d^{2}X}{dt^{2}}}{\vec {i}}=a_{x}{\vec {i}}}
Y
→
=
Y
j
→
{\displaystyle {\vec {Y}}=Y{\vec {j}}}
d
d
t
Y
→
=
d
Y
d
t
j
→
=
v
y
j
→
{\displaystyle {\frac {d}{dt}}{\vec {Y}}={\frac {dY}{dt}}{\vec {j}}=v_{y}{\vec {j}}}
d
2
d
t
2
Y
→
=
d
2
Y
d
t
2
j
→
=
a
y
j
→
{\displaystyle {\frac {d^{2}}{dt^{2}}}{\vec {Y}}={\frac {d^{2}Y}{dt^{2}}}{\vec {j}}=a_{y}{\vec {j}}}
Z
→
=
Z
k
→
{\displaystyle {\vec {Z}}=Z{\vec {k}}}
d
d
t
Z
→
=
d
Z
d
t
k
→
=
v
z
k
→
{\displaystyle {\frac {d}{dt}}{\vec {Z}}={\frac {dZ}{dt}}{\vec {k}}=v_{z}{\vec {k}}}
d
2
d
t
2
Z
→
=
d
2
Z
d
t
2
k
→
=
a
z
k
→
{\displaystyle {\frac {d^{2}}{dt^{2}}}{\vec {Z}}={\frac {d^{2}Z}{dt^{2}}}{\vec {k}}=a_{z}{\vec {k}}}
R
→
=
R
r
→
{\displaystyle {\vec {R}}=R{\vec {r}}}
d
d
t
R
→
{\displaystyle {\frac {d}{dt}}{\vec {R}}}
R
d
d
t
r
→
+
r
→
d
d
t
R
=
R
d
d
t
r
→
{\displaystyle R{\frac {d}{dt}}{\vec {r}}+{\vec {r}}{\frac {d}{dt}}R=R{\frac {d}{dt}}{\vec {r}}}
d
2
d
t
2
R
→
{\displaystyle {\frac {d^{2}}{dt^{2}}}{\vec {R}}}
R
d
2
d
t
2
r
→
+
r
→
d
2
d
t
2
R
=
R
d
2
d
t
2
r
→
{\displaystyle R{\frac {d^{2}}{dt^{2}}}{\vec {r}}+{\vec {r}}{\frac {d^{2}}{dt^{2}}}R=R{\frac {d^{2}}{dt^{2}}}{\vec {r}}}
R
→
=
X
→
+
Y
→
{\displaystyle {\vec {R}}={\vec {X}}+{\vec {Y}}}
d
d
t
R
→
=
d
d
t
(
X
→
+
Y
→
)
{\displaystyle {\frac {d}{dt}}{\vec {R}}={\frac {d}{dt}}({\vec {X}}+{\vec {Y}})}
d
X
d
t
i
→
+
d
Y
d
t
j
→
=
v
x
i
→
+
v
y
j
→
{\displaystyle {\frac {dX}{dt}}{\vec {i}}+{\frac {dY}{dt}}{\vec {j}}=v_{x}{\vec {i}}+v_{y}{\vec {j}}}
d
2
d
t
2
R
→
=
d
2
d
t
2
(
X
→
+
Y
→
)
{\displaystyle {\frac {d^{2}}{dt^{2}}}{\vec {R}}={\frac {d^{2}}{dt^{2}}}({\vec {X}}+{\vec {Y}})}
d
2
X
d
t
2
i
→
+
d
2
Y
d
t
2
j
→
=
a
x
i
→
+
a
y
j
→
{\displaystyle {\frac {d^{2}X}{dt^{2}}}{\vec {i}}+{\frac {d^{2}Y}{dt^{2}}}{\vec {j}}=a_{x}{\vec {i}}+a_{y}{\vec {j}}}
Operator notation [ sửa ] Gradient [ sửa ] Bản mẫu:Main
For a function
f
(
x
,
y
,
z
)
{\displaystyle f(x,y,z)}
Cartesian coordinate variables, the gradient is the vector field:
grad
(
f
)
=
∇
f
=
(
∂
∂
x
,
∂
∂
y
,
∂
∂
z
)
f
=
∂
f
∂
x
i
+
∂
f
∂
y
j
+
∂
f
∂
z
k
{\displaystyle \operatorname {grad} (f)=\nabla f={\begin{pmatrix}\displaystyle {\frac {\partial }{\partial x}},\ {\frac {\partial }{\partial y}},\ {\frac {\partial }{\partial z}}\end{pmatrix}}f={\frac {\partial f}{\partial x}}\mathbf {i} +{\frac {\partial f}{\partial y}}\mathbf {j} +{\frac {\partial f}{\partial z}}\mathbf {k} }
where i , j , k are the standard unit vectors for the x , y , z -axes. More generally, for a function of n variables
ψ
(
x
1
,
…
,
x
n
)
{\displaystyle \psi (x_{1},\ldots ,x_{n})}
scalar field, the gradient is the vector field :
∇
ψ
=
(
∂
∂
x
1
,
…
,
∂
∂
x
n
)
ψ
=
∂
ψ
∂
x
1
e
1
+
⋯
+
∂
ψ
∂
x
n
e
n
{\displaystyle \nabla \psi ={\begin{pmatrix}\displaystyle {\frac {\partial }{\partial x_{1}}},\ldots ,{\frac {\partial }{\partial x_{n}}}\end{pmatrix}}\psi ={\frac {\partial \psi }{\partial x_{1}}}\mathbf {e} _{1}+\dots +{\frac {\partial \psi }{\partial x_{n}}}\mathbf {e} _{n}}
where
e
i
(
i
=
1
,
2
,
.
.
.
,
n
)
{\displaystyle \mathbf {e} _{i}\,(i=1,2,...,n)}
are mutually orthogonal unit vectors.
As the name implies, the gradient is proportional to, and points in the direction of, the function's most rapid (positive) change.
For a vector field
A
=
(
A
1
,
…
,
A
n
)
{\displaystyle \mathbf {A} =\left(A_{1},\ldots ,A_{n}\right)}
total derivative is the n × n Jacobian matrix :
J
A
=
d
A
=
(
∇
A
)
T
=
(
∂
A
i
∂
x
j
)
i
j
.
{\displaystyle \mathbf {J} _{\mathbf {A} }=d\mathbf {A} =(\nabla \!\mathbf {A} )^{\textsf {T}}=\left({\frac {\partial A_{i}}{\partial x_{j}}}\right)_{\!ij}.}
For a tensor field
T
{\displaystyle \mathbf {T} }
k , the gradient
grad
(
T
)
=
d
T
=
(
∇
T
)
T
{\displaystyle \operatorname {grad} (\mathbf {T} )=d\mathbf {T} =(\nabla \mathbf {T} )^{\textsf {T}}}
k + 1.
For a tensor field
T
{\displaystyle \mathbf {T} }
k > 0, the tensor field
∇
T
{\displaystyle \nabla \mathbf {T} }
k + 1 is defined by the recursive relation
(
∇
T
)
⋅
C
=
∇
(
T
⋅
C
)
{\displaystyle (\nabla \mathbf {T} )\cdot \mathbf {C} =\nabla (\mathbf {T} \cdot \mathbf {C} )}
where
C
{\displaystyle \mathbf {C} }
is an arbitrary constant vector.
Divergence [ sửa ] Bản mẫu:Main
In Cartesian coordinates, the divergence of a continuously differentiable vector field
F
=
F
x
i
+
F
y
j
+
F
z
k
{\displaystyle \mathbf {F} =F_{x}\mathbf {i} +F_{y}\mathbf {j} +F_{z}\mathbf {k} }
div
F
=
∇
⋅
F
=
(
∂
∂
x
,
∂
∂
y
,
∂
∂
z
)
⋅
(
F
x
,
F
y
,
F
z
)
=
∂
F
x
∂
x
+
∂
F
y
∂
y
+
∂
F
z
∂
z
.
{\displaystyle \operatorname {div} \mathbf {F} =\nabla \cdot \mathbf {F} ={\begin{pmatrix}\displaystyle {\frac {\partial }{\partial x}},\ {\frac {\partial }{\partial y}},\ {\frac {\partial }{\partial z}}\end{pmatrix}}\cdot {\begin{pmatrix}F_{x},\ F_{y},\ F_{z}\end{pmatrix}}={\frac {\partial F_{x}}{\partial x}}+{\frac {\partial F_{y}}{\partial y}}+{\frac {\partial F_{z}}{\partial z}}.}
As the name implies, the divergence is a (local) measure of the degree to which vectors in the field diverge.
The divergence of a tensor field
T
{\displaystyle \mathbf {T} }
k is written as
div
(
T
)
=
∇
⋅
T
{\displaystyle \operatorname {div} (\mathbf {T} )=\nabla \cdot \mathbf {T} }
contraction of a tensor field of order k − 1. Specifically, the divergence of a vector is a scalar. The divergence of a higher-order tensor field may be found by decomposing the tensor field into a sum of outer products and using the identity,
∇
⋅
(
A
⊗
T
)
=
T
(
∇
⋅
A
)
+
(
A
⋅
∇
)
T
{\displaystyle \nabla \cdot \left(\mathbf {A} \otimes \mathbf {T} \right)=\mathbf {T} (\nabla \cdot \mathbf {A} )+(\mathbf {A} \cdot \nabla )\mathbf {T} }
where
A
⋅
∇
{\displaystyle \mathbf {A} \cdot \nabla }
is the
directional derivative in the direction of
A
{\displaystyle \mathbf {A} }
multiplied by its magnitude. Specifically, for the outer product of two vectors,
∇
⋅
(
A
B
T
)
=
B
(
∇
⋅
A
)
+
(
A
⋅
∇
)
B
.
{\displaystyle \nabla \cdot \left(\mathbf {A} \mathbf {B} ^{\textsf {T}}\right)=\mathbf {B} (\nabla \cdot \mathbf {A} )+(\mathbf {A} \cdot \nabla )\mathbf {B} .}
For a tensor field
T
{\displaystyle \mathbf {T} }
k > 1, the tensor field
∇
⋅
T
{\displaystyle \nabla \cdot \mathbf {T} }
k − 1 is defined by the recursive relation
(
∇
⋅
T
)
⋅
C
=
∇
⋅
(
T
⋅
C
)
{\displaystyle (\nabla \cdot \mathbf {T} )\cdot \mathbf {C} =\nabla \cdot (\mathbf {T} \cdot \mathbf {C} )}
where
C
{\displaystyle \mathbf {C} }
is an arbitrary constant vector.
Bản mẫu:Main
In Cartesian coordinates, for
F
=
F
x
i
+
F
y
j
+
F
z
k
{\displaystyle \mathbf {F} =F_{x}\mathbf {i} +F_{y}\mathbf {j} +F_{z}\mathbf {k} }
curl
F
=
∇
×
F
=
(
∂
∂
x
,
∂
∂
y
,
∂
∂
z
)
×
(
F
x
,
F
y
,
F
z
)
=
|
i
j
k
∂
∂
x
∂
∂
y
∂
∂
z
F
x
F
y
F
z
|
=
(
∂
F
z
∂
y
−
∂
F
y
∂
z
)
i
+
(
∂
F
x
∂
z
−
∂
F
z
∂
x
)
j
+
(
∂
F
y
∂
x
−
∂
F
x
∂
y
)
k
{\displaystyle {\begin{aligned}\operatorname {curl} \mathbf {F} &=\nabla \times \mathbf {F} ={\begin{pmatrix}\displaystyle {\frac {\partial }{\partial x}},\ {\frac {\partial }{\partial y}},\ {\frac {\partial }{\partial z}}\end{pmatrix}}\times {\begin{pmatrix}F_{x},\ F_{y},\ F_{z}\end{pmatrix}}={\begin{vmatrix}\mathbf {i} &\mathbf {j} &\mathbf {k} \\{\frac {\partial }{\partial x}}&{\frac {\partial }{\partial y}}&{\frac {\partial }{\partial z}}\\F_{x}&F_{y}&F_{z}\end{vmatrix}}\\[1em]&=\left({\frac {\partial F_{z}}{\partial y}}-{\frac {\partial F_{y}}{\partial z}}\right)\mathbf {i} +\left({\frac {\partial F_{x}}{\partial z}}-{\frac {\partial F_{z}}{\partial x}}\right)\mathbf {j} +\left({\frac {\partial F_{y}}{\partial x}}-{\frac {\partial F_{x}}{\partial y}}\right)\mathbf {k} \end{aligned}}}
where
i ,
j , and
k are the
unit vectors for the
x -,
y -, and
z -axes, respectively.
As the name implies the curl is a measure of how much nearby vectors tend in a circular direction.
In Einstein notation , the vector field
F
=
(
F
1
,
F
2
,
F
3
)
{\displaystyle \mathbf {F} ={\begin{pmatrix}F_{1},\ F_{2},\ F_{3}\end{pmatrix}}}
∇
×
F
=
ε
i
j
k
e
i
∂
F
k
∂
x
j
{\displaystyle \nabla \times \mathbf {F} =\varepsilon ^{ijk}\mathbf {e} _{i}{\frac {\partial F_{k}}{\partial x_{j}}}}
where
ε
{\displaystyle \varepsilon }
= ±1 or 0 is the
Levi-Civita parity symbol .
For a tensor field
T
{\displaystyle \mathbf {T} }
k > 1, the tensor field
∇
×
T
{\displaystyle \nabla \times \mathbf {T} }
k is defined by the recursive relation
(
∇
×
T
)
⋅
C
=
∇
×
(
T
⋅
C
)
{\displaystyle (\nabla \times \mathbf {T} )\cdot \mathbf {C} =\nabla \times (\mathbf {T} \cdot \mathbf {C} )}
where
C
{\displaystyle \mathbf {C} }
is an arbitrary constant vector.
A tensor field of order greater than one may be decomposed into a sum of outer products , and then the following identity may be used:
∇
×
(
A
⊗
T
)
=
(
∇
×
A
)
⊗
T
−
A
×
(
∇
T
)
.
{\displaystyle \nabla \times \left(\mathbf {A} \otimes \mathbf {T} \right)=(\nabla \times \mathbf {A} )\otimes \mathbf {T} -\mathbf {A} \times (\nabla \mathbf {T} ).}
Specifically, for the outer product of two vectors,
∇
×
(
A
B
T
)
=
(
∇
×
A
)
B
T
−
A
×
(
∇
B
)
.
{\displaystyle \nabla \times \left(\mathbf {A} \mathbf {B} ^{\textsf {T}}\right)=(\nabla \times \mathbf {A} )\mathbf {B} ^{\textsf {T}}-\mathbf {A} \times (\nabla \mathbf {B} ).}
Laplacian [ sửa ] Bản mẫu:Main
In Cartesian coordinates , the Laplacian of a function
f
(
x
,
y
,
z
)
{\displaystyle f(x,y,z)}
Δ
f
=
∇
2
f
=
(
∇
⋅
∇
)
f
=
∂
2
f
∂
x
2
+
∂
2
f
∂
y
2
+
∂
2
f
∂
z
2
.
{\displaystyle \Delta f=\nabla ^{2}\!f=(\nabla \cdot \nabla )f={\frac {\partial ^{2}\!f}{\partial x^{2}}}+{\frac {\partial ^{2}\!f}{\partial y^{2}}}+{\frac {\partial ^{2}\!f}{\partial z^{2}}}.}
The Laplacian is a measure of how much a function is changing over a small sphere centered at the point.
When the Laplacian is equal to 0, the function is called a harmonic function . That is,
Δ
f
=
0.
{\displaystyle \Delta f=0.}
For a tensor field ,
T
{\displaystyle \mathbf {T} }
Δ
T
=
∇
2
T
=
(
∇
⋅
∇
)
T
{\displaystyle \Delta \mathbf {T} =\nabla ^{2}\mathbf {T} =(\nabla \cdot \nabla )\mathbf {T} }
and is a tensor field of the same order.
For a tensor field
T
{\displaystyle \mathbf {T} }
k > 0, the tensor field
∇
2
T
{\displaystyle \nabla ^{2}\mathbf {T} }
k is defined by the recursive relation
(
∇
2
T
)
⋅
C
=
∇
2
(
T
⋅
C
)
{\displaystyle \left(\nabla ^{2}\mathbf {T} \right)\cdot \mathbf {C} =\nabla ^{2}(\mathbf {T} \cdot \mathbf {C} )}
where
C
{\displaystyle \mathbf {C} }
is an arbitrary constant vector.
Special notations [ sửa ] In Feynman subscript notation ,
∇
B
(
A
⋅
B
)
=
A
×
(
∇
×
B
)
+
(
A
⋅
∇
)
B
{\displaystyle \nabla _{\mathbf {B} }\!\left(\mathbf {A{\cdot }B} \right)=\mathbf {A} {\times }\!\left(\nabla {\times }\mathbf {B} \right)+\left(\mathbf {A} {\cdot }\nabla \right)\mathbf {B} }
where the notation ∇
B means the subscripted gradient operates on only the factor
B .
[1] [2] Less general but similar is the Hestenes overdot notation in geometric algebra .[3]
∇
˙
(
A
⋅
B
˙
)
=
A
×
(
∇
×
B
)
+
(
A
⋅
∇
)
B
{\displaystyle {\dot {\nabla }}\left(\mathbf {A} {\cdot }{\dot {\mathbf {B} }}\right)=\mathbf {A} {\times }\!\left(\nabla {\times }\mathbf {B} \right)+\left(\mathbf {A} {\cdot }\nabla \right)\mathbf {B} }
where overdots define the scope of the vector derivative. The dotted vector, in this case
B , is differentiated, while the (undotted)
A is held constant.
For the remainder of this article, Feynman subscript notation will be used where appropriate.
First derivative identities [ sửa ] For scalar fields
ψ
{\displaystyle \psi }
ϕ
{\displaystyle \phi }
A
{\displaystyle \mathbf {A} }
B
{\displaystyle \mathbf {B} }
Distributive properties [ sửa ]
∇
(
ψ
+
ϕ
)
=
∇
ψ
+
∇
ϕ
∇
(
A
+
B
)
=
∇
A
+
∇
B
∇
⋅
(
A
+
B
)
=
∇
⋅
A
+
∇
⋅
B
∇
×
(
A
+
B
)
=
∇
×
A
+
∇
×
B
{\displaystyle {\begin{aligned}\nabla (\psi +\phi )&=\nabla \psi +\nabla \phi \\\nabla (\mathbf {A} +\mathbf {B} )&=\nabla \mathbf {A} +\nabla \mathbf {B} \\\nabla \cdot (\mathbf {A} +\mathbf {B} )&=\nabla \cdot \mathbf {A} +\nabla \cdot \mathbf {B} \\\nabla \times (\mathbf {A} +\mathbf {B} )&=\nabla \times \mathbf {A} +\nabla \times \mathbf {B} \end{aligned}}}
First derivative associative properties [ sửa ]
(
A
⋅
∇
)
ψ
=
A
⋅
(
∇
ψ
)
(
A
⋅
∇
)
B
=
A
⋅
(
∇
B
)
(
A
×
∇
)
ψ
=
A
×
(
∇
ψ
)
(
A
×
∇
)
B
=
A
×
(
∇
B
)
{\displaystyle {\begin{aligned}(\mathbf {A} \cdot \nabla )\psi &=\mathbf {A} \cdot (\nabla \psi )\\(\mathbf {A} \cdot \nabla )\mathbf {B} &=\mathbf {A} \cdot (\nabla \mathbf {B} )\\(\mathbf {A} \times \nabla )\psi &=\mathbf {A} \times (\nabla \psi )\\(\mathbf {A} \times \nabla )\mathbf {B} &=\mathbf {A} \times (\nabla \mathbf {B} )\end{aligned}}}
Product rule for multiplication by a scalar [ sửa ] We have the following generalizations of the product rule in single-variable calculus .
∇
(
ψ
ϕ
)
=
ϕ
∇
ψ
+
ψ
∇
ϕ
∇
(
ψ
A
)
=
(
∇
ψ
)
A
T
+
ψ
∇
A
=
∇
ψ
⊗
A
+
ψ
∇
A
∇
⋅
(
ψ
A
)
=
ψ
∇
⋅
A
+
(
∇
ψ
)
⋅
A
∇
×
(
ψ
A
)
=
ψ
∇
×
A
+
(
∇
ψ
)
×
A
∇
2
(
ψ
ϕ
)
=
ψ
∇
2
ϕ
+
2
∇
ψ
⋅
∇
ϕ
+
ϕ
∇
2
ψ
{\displaystyle {\begin{aligned}\nabla (\psi \phi )&=\phi \,\nabla \psi +\psi \,\nabla \phi \\\nabla (\psi \mathbf {A} )&=(\nabla \psi )\mathbf {A} ^{\textsf {T}}+\psi \nabla \mathbf {A} \ =\ \nabla \psi \otimes \mathbf {A} +\psi \,\nabla \mathbf {A} \\\nabla \cdot (\psi \mathbf {A} )&=\psi \,\nabla {\cdot }\mathbf {A} +(\nabla \psi )\,{\cdot }\mathbf {A} \\\nabla {\times }(\psi \mathbf {A} )&=\psi \,\nabla {\times }\mathbf {A} +(\nabla \psi ){\times }\mathbf {A} \\\nabla ^{2}(\psi \phi )&=\psi \,\nabla ^{2\!}\phi +2\,\nabla \!\psi \cdot \!\nabla \phi +\phi \,\nabla ^{2\!}\psi \end{aligned}}}
Quotient rule for division by a scalar [ sửa ]
∇
(
ψ
ϕ
)
=
ϕ
∇
ψ
−
ψ
∇
ϕ
ϕ
2
∇
(
A
ϕ
)
=
ϕ
∇
A
−
∇
ϕ
⊗
A
ϕ
2
∇
⋅
(
A
ϕ
)
=
ϕ
∇
⋅
A
−
∇
ϕ
⋅
A
ϕ
2
∇
×
(
A
ϕ
)
=
ϕ
∇
×
A
−
∇
ϕ
×
A
ϕ
2
∇
2
(
ψ
ϕ
)
=
ϕ
∇
2
ψ
−
2
ϕ
∇
(
ψ
ϕ
)
⋅
∇
ϕ
−
ψ
∇
2
ϕ
ϕ
2
{\displaystyle {\begin{aligned}\nabla \left({\frac {\psi }{\phi }}\right)&={\frac {\phi \,\nabla \psi -\psi \,\nabla \phi }{\phi ^{2}}}\\[1em]\nabla \left({\frac {\mathbf {A} }{\phi }}\right)&={\frac {\phi \,\nabla \mathbf {A} -\nabla \phi \otimes \mathbf {A} }{\phi ^{2}}}\\[1em]\nabla \cdot \left({\frac {\mathbf {A} }{\phi }}\right)&={\frac {\phi \,\nabla {\cdot }\mathbf {A} -\nabla \!\phi \cdot \mathbf {A} }{\phi ^{2}}}\\[1em]\nabla \times \left({\frac {\mathbf {A} }{\phi }}\right)&={\frac {\phi \,\nabla {\times }\mathbf {A} -\nabla \!\phi \,{\times }\,\mathbf {A} }{\phi ^{2}}}\\[1em]\nabla ^{2}\left({\frac {\psi }{\phi }}\right)&={\frac {\phi \,\nabla ^{2\!}\psi -2\,\phi \,\nabla \!\left({\frac {\psi }{\phi }}\right)\cdot \!\nabla \phi -\psi \,\nabla ^{2\!}\phi }{\phi ^{2}}}\end{aligned}}}
Chain rule [ sửa ] Let
f
(
x
)
{\displaystyle f(x)}
r
(
t
)
=
(
x
1
(
t
)
,
…
,
x
n
(
t
)
)
{\displaystyle \mathbf {r} (t)=(x_{1}(t),\ldots ,x_{n}(t))}
parametrized curve,
ϕ
:
R
n
→
R
{\displaystyle \phi \!:\mathbb {R} ^{n}\to \mathbb {R} }
A
:
R
n
→
R
n
{\displaystyle \mathbf {A} \!:\mathbb {R} ^{n}\to \mathbb {R} ^{n}}
chain rule .
∇
(
f
∘
ϕ
)
=
(
f
′
∘
ϕ
)
∇
ϕ
(
r
∘
f
)
′
=
(
r
′
∘
f
)
f
′
(
ϕ
∘
r
)
′
=
(
∇
ϕ
∘
r
)
⋅
r
′
(
A
∘
r
)
′
=
r
′
⋅
(
∇
A
∘
r
)
∇
(
ϕ
∘
A
)
=
(
∇
A
)
⋅
(
∇
ϕ
∘
A
)
∇
⋅
(
r
∘
ϕ
)
=
∇
ϕ
⋅
(
r
′
∘
ϕ
)
∇
×
(
r
∘
ϕ
)
=
∇
ϕ
×
(
r
′
∘
ϕ
)
{\displaystyle {\begin{aligned}\nabla (f\circ \phi )&=\left(f'\circ \phi \right)\nabla \phi \\(\mathbf {r} \circ f)'&=(\mathbf {r} '\circ f)f'\\(\phi \circ \mathbf {r} )'&=(\nabla \phi \circ \mathbf {r} )\cdot \mathbf {r} '\\(\mathbf {A} \circ \mathbf {r} )'&=\mathbf {r} '\cdot (\nabla \mathbf {A} \circ \mathbf {r} )\\\nabla (\phi \circ \mathbf {A} )&=(\nabla \mathbf {A} )\cdot (\nabla \phi \circ \mathbf {A} )\\\nabla \cdot (\mathbf {r} \circ \phi )&=\nabla \phi \cdot (\mathbf {r} '\circ \phi )\\\nabla \times (\mathbf {r} \circ \phi )&=\nabla \phi \times (\mathbf {r} '\circ \phi )\end{aligned}}}
For a vector transformation
x
:
R
n
→
R
n
{\displaystyle \mathbf {x} \!:\mathbb {R} ^{n}\to \mathbb {R} ^{n}}
∇
⋅
(
A
∘
x
)
=
t
r
(
(
∇
x
)
⋅
(
∇
A
∘
x
)
)
{\displaystyle \nabla \cdot (\mathbf {A} \circ \mathbf {x} )=\mathrm {tr} \left((\nabla \mathbf {x} )\cdot (\nabla \mathbf {A} \circ \mathbf {x} )\right)}
Here we take the trace of the dot product of two second-order tensors, which corresponds to the product of their matrices.
Dot product rule [ sửa ]
∇
(
A
⋅
B
)
=
(
A
⋅
∇
)
B
+
(
B
⋅
∇
)
A
+
A
×
(
∇
×
B
)
+
B
×
(
∇
×
A
)
=
A
⋅
J
B
+
B
⋅
J
A
=
(
∇
B
)
⋅
A
+
(
∇
A
)
⋅
B
{\displaystyle {\begin{aligned}\nabla (\mathbf {A} \cdot \mathbf {B} )&\ =\ (\mathbf {A} \cdot \nabla )\mathbf {B} \,+\,(\mathbf {B} \cdot \nabla )\mathbf {A} \,+\,\mathbf {A} {\times }(\nabla {\times }\mathbf {B} )\,+\,\mathbf {B} {\times }(\nabla {\times }\mathbf {A} )\\&\ =\ \mathbf {A} \cdot \mathbf {J} _{\mathbf {B} }+\mathbf {B} \cdot \mathbf {J} _{\mathbf {A} }\ =\ (\nabla \mathbf {B} )\cdot \mathbf {A} \,+\,(\nabla \mathbf {A} )\cdot \mathbf {B} \end{aligned}}}
where
J
A
=
(
∇
A
)
T
=
(
∂
A
i
/
∂
x
j
)
i
j
{\displaystyle \mathbf {J} _{\mathbf {A} }=(\nabla \!\mathbf {A} )^{\textsf {T}}=(\partial A_{i}/\partial x_{j})_{ij}}
Jacobian matrix of the vector field
A
=
(
A
1
,
…
,
A
n
)
{\displaystyle \mathbf {A} =(A_{1},\ldots ,A_{n})}
Alternatively, using Feynman subscript notation,
∇
(
A
⋅
B
)
=
∇
A
(
A
⋅
B
)
+
∇
B
(
A
⋅
B
)
.
{\displaystyle \nabla (\mathbf {A} \cdot \mathbf {B} )=\nabla _{\mathbf {A} }(\mathbf {A} \cdot \mathbf {B} )+\nabla _{\mathbf {B} }(\mathbf {A} \cdot \mathbf {B} )\ .}
See these notes.[4]
As a special case, when Bản mẫu:Math ,
1
2
∇
(
A
⋅
A
)
=
A
⋅
J
A
=
(
∇
A
)
⋅
A
=
(
A
⋅
∇
)
A
+
A
×
(
∇
×
A
)
=
A
∇
A
.
{\displaystyle {\tfrac {1}{2}}\nabla \left(\mathbf {A} \cdot \mathbf {A} \right)\ =\ \mathbf {A} \cdot \mathbf {J} _{\mathbf {A} }\ =\ (\nabla \mathbf {A} )\cdot \mathbf {A} \ =\ (\mathbf {A} {\cdot }\nabla )\mathbf {A} \,+\,\mathbf {A} {\times }(\nabla {\times }\mathbf {A} )\ =\ A\nabla A.}
The generalization of the dot product formula to Riemannian manifolds is a defining property of a Riemannian connection , which differentiates a vector field to give a vector-valued 1-form .
Cross product rule [ sửa ]
∇
⋅
(
A
×
B
)
=
(
∇
×
A
)
⋅
B
−
A
⋅
(
∇
×
B
)
∇
×
(
A
×
B
)
=
A
(
∇
⋅
B
)
−
B
(
∇
⋅
A
)
+
(
B
⋅
∇
)
A
−
(
A
⋅
∇
)
B
=
A
(
∇
⋅
B
)
+
(
B
⋅
∇
)
A
−
(
B
(
∇
⋅
A
)
+
(
A
⋅
∇
)
B
)
=
∇
⋅
(
B
A
T
)
−
∇
⋅
(
A
B
T
)
=
∇
⋅
(
B
A
T
−
A
B
T
)
A
×
(
∇
×
B
)
=
∇
B
(
A
⋅
B
)
−
(
A
⋅
∇
)
B
=
A
⋅
J
B
−
(
A
⋅
∇
)
B
=
(
∇
B
)
⋅
A
−
A
⋅
(
∇
B
)
=
A
⋅
(
J
B
−
J
B
T
)
(
A
×
∇
)
×
B
=
(
∇
B
)
⋅
A
−
A
(
∇
⋅
B
)
=
A
×
(
∇
×
B
)
+
A
⋅
(
∇
B
)
−
A
(
∇
⋅
B
)
(
A
×
∇
)
⋅
B
=
A
⋅
(
∇
×
B
)
{\displaystyle {\begin{aligned}\nabla \cdot (\mathbf {A} \times \mathbf {B} )&\ =\ (\nabla {\times }\mathbf {A} )\cdot \mathbf {B} \,-\,\mathbf {A} \cdot (\nabla {\times }\mathbf {B} )\\[5pt]\nabla \times (\mathbf {A} \times \mathbf {B} )&\ =\ \mathbf {A} (\nabla {\cdot }\mathbf {B} )\,-\,\mathbf {B} (\nabla {\cdot }\mathbf {A} )\,+\,(\mathbf {B} {\cdot }\nabla )\mathbf {A} \,-\,(\mathbf {A} {\cdot }\nabla )\mathbf {B} \\[2pt]&\ =\ \mathbf {A} (\nabla {\cdot }\mathbf {B} )\,+\,(\mathbf {B} {\cdot }\nabla )\mathbf {A} \,-\,(\mathbf {B} (\nabla {\cdot }\mathbf {A} )\,+\,(\mathbf {A} {\cdot }\nabla )\mathbf {B} )\\[2pt]&\ =\ \nabla {\cdot }\left(\mathbf {B} \mathbf {A} ^{\textsf {T}}\right)\,-\,\nabla {\cdot }\left(\mathbf {A} \mathbf {B} ^{\textsf {T}}\right)\\[2pt]&\ =\ \nabla {\cdot }\left(\mathbf {B} \mathbf {A} ^{\textsf {T}}\,-\,\mathbf {A} \mathbf {B} ^{\textsf {T}}\right)\\[5pt]\mathbf {A} \times (\nabla \times \mathbf {B} )&\ =\ \nabla _{\mathbf {B} }(\mathbf {A} {\cdot }\mathbf {B} )\,-\,(\mathbf {A} {\cdot }\nabla )\mathbf {B} \\[2pt]&\ =\ \mathbf {A} \cdot \mathbf {J} _{\mathbf {B} }\,-\,(\mathbf {A} {\cdot }\nabla )\mathbf {B} \\[2pt]&\ =\ (\nabla \mathbf {B} )\cdot \mathbf {A} \,-\,\mathbf {A} \cdot (\nabla \mathbf {B} )\\[2pt]&\ =\ \mathbf {A} \cdot (\mathbf {J} _{\mathbf {B} }\,-\,\mathbf {J} _{\mathbf {B} }^{\textsf {T}})\\[5pt](\mathbf {A} \times \nabla )\times \mathbf {B} &\ =\ (\nabla \mathbf {B} )\cdot \mathbf {A} \,-\,\mathbf {A} (\nabla {\cdot }\mathbf {B} )\\[2pt]&\ =\ \mathbf {A} \times (\nabla \times \mathbf {B} )\,+\,\mathbf {A} {\cdot }(\nabla \mathbf {B} )\,-\,\mathbf {A} (\nabla {\cdot }\mathbf {B} )\\[5pt](\mathbf {A} \times \nabla )\cdot \mathbf {B} &\ =\ \mathbf {A} \cdot (\nabla {\times }\mathbf {B} )\end{aligned}}}
J
B
−
J
B
T
{\displaystyle \mathbf {J} _{\mathbf {B} }\,-\,\mathbf {J} _{\mathbf {B} }^{\textsf {T}}}
Second derivative identities [ sửa ] Divergence of curl is zero [ sửa ] The divergence of the curl of any continuously twice-differentiable vector field A is always zero:
∇
⋅
(
∇
×
A
)
=
0
{\displaystyle \nabla \cdot (\nabla \times \mathbf {A} )=0}
This is a special case of the vanishing of the square of the exterior derivative in the De Rham chain complex .
Divergence of gradient is Laplacian [ sửa ] The Laplacian of a scalar field is the divergence of its gradient:
Δ
ψ
=
∇
2
ψ
=
∇
⋅
(
∇
ψ
)
{\displaystyle \Delta \psi =\nabla ^{2}\psi =\nabla \cdot (\nabla \psi )}
The result is a scalar quantity.
Divergence of divergence is not defined [ sửa ] The divergence of a vector field A is a scalar, and the divergence of a scalar quantity is undefined. Therefore,
∇
⋅
(
∇
⋅
A
)
is undefined.
{\displaystyle \nabla \cdot (\nabla \cdot \mathbf {A} ){\text{ is undefined.}}}
Curl of gradient is zero [ sửa ] The curl of the gradient of any continuously twice-differentiable scalar field
φ
{\displaystyle \varphi }
differentiability class
C
2
{\displaystyle C^{2}}
zero vector :
∇
×
(
∇
φ
)
=
0
.
{\displaystyle \nabla \times (\nabla \varphi )=\mathbf {0} .}
It can be easily proved by expressing
∇
×
(
∇
φ
)
{\displaystyle \nabla \times (\nabla \varphi )}
Cartesian coordinate system with Schwarz's theorem (also called Clairaut's theorem on equality of mixed partials). This result is a special case of the vanishing of the square of the exterior derivative in the De Rham chain complex .
Curl of curl [ sửa ]
∇
×
(
∇
×
A
)
=
∇
(
∇
⋅
A
)
−
∇
2
A
{\displaystyle \nabla \times \left(\nabla \times \mathbf {A} \right)\ =\ \nabla (\nabla {\cdot }\mathbf {A} )\,-\,\nabla ^{2\!}\mathbf {A} }
Here ∇2 is the vector Laplacian operating on the vector field A .
Curl of divergence is not defined [ sửa ] The divergence of a vector field A is a scalar, and the curl of a scalar quantity is undefined. Therefore,
∇
×
(
∇
⋅
A
)
is undefined.
{\displaystyle \nabla \times (\nabla \cdot \mathbf {A} ){\text{ is undefined.}}}
Second derivative associative properties [ sửa ]
(
∇
⋅
∇
)
ψ
=
∇
⋅
(
∇
ψ
)
=
∇
2
ψ
(
∇
⋅
∇
)
A
=
∇
⋅
(
∇
A
)
=
∇
2
A
(
∇
×
∇
)
ψ
=
∇
×
(
∇
ψ
)
=
0
(
∇
×
∇
)
A
=
∇
×
(
∇
A
)
=
0
{\displaystyle {\begin{aligned}(\nabla \cdot \nabla )\psi &=\nabla \cdot (\nabla \psi )=\nabla ^{2}\psi \\(\nabla \cdot \nabla )\mathbf {A} &=\nabla \cdot (\nabla \mathbf {A} )=\nabla ^{2}\mathbf {A} \\(\nabla \times \nabla )\psi &=\nabla \times (\nabla \psi )=\mathbf {0} \\(\nabla \times \nabla )\mathbf {A} &=\nabla \times (\nabla \mathbf {A} )=\mathbf {0} \end{aligned}}}
DCG chart: Some rules for second derivatives. A mnemonic [ sửa ] The figure to the right is a mnemonic for some of these identities. The abbreviations used are:
D: divergence,
C: curl,
G: gradient,
L: Laplacian,
CC: curl of curl. Each arrow is labeled with the result of an identity, specifically, the result of applying the operator at the arrow's tail to the operator at its head. The blue circle in the middle means curl of curl exists, whereas the other two red circles (dashed) mean that DD and GG do not exist.
Summary of important identities [ sửa ] Differentiation [ sửa ] Gradient [ sửa ]
∇
(
ψ
+
ϕ
)
=
∇
ψ
+
∇
ϕ
{\displaystyle \nabla (\psi +\phi )=\nabla \psi +\nabla \phi }
∇
(
ψ
ϕ
)
=
ϕ
∇
ψ
+
ψ
∇
ϕ
{\displaystyle \nabla (\psi \phi )=\phi \nabla \psi +\psi \nabla \phi }
∇
(
ψ
A
)
=
∇
ψ
⊗
A
+
ψ
∇
A
{\displaystyle \nabla (\psi \mathbf {A} )=\nabla \psi \otimes \mathbf {A} +\psi \nabla \mathbf {A} }
∇
(
A
⋅
B
)
=
(
A
⋅
∇
)
B
+
(
B
⋅
∇
)
A
+
A
×
(
∇
×
B
)
+
B
×
(
∇
×
A
)
{\displaystyle \nabla (\mathbf {A} \cdot \mathbf {B} )=(\mathbf {A} \cdot \nabla )\mathbf {B} +(\mathbf {B} \cdot \nabla )\mathbf {A} +\mathbf {A} \times (\nabla \times \mathbf {B} )+\mathbf {B} \times (\nabla \times \mathbf {A} )}
Divergence [ sửa ]
∇
⋅
(
A
+
B
)
=
∇
⋅
A
+
∇
⋅
B
{\displaystyle \nabla \cdot (\mathbf {A} +\mathbf {B} )=\nabla \cdot \mathbf {A} +\nabla \cdot \mathbf {B} }
∇
⋅
(
ψ
A
)
=
ψ
∇
⋅
A
+
A
⋅
∇
ψ
{\displaystyle \nabla \cdot \left(\psi \mathbf {A} \right)=\psi \nabla \cdot \mathbf {A} +\mathbf {A} \cdot \nabla \psi }
∇
⋅
(
A
×
B
)
=
(
∇
×
A
)
⋅
B
−
(
∇
×
B
)
⋅
A
{\displaystyle \nabla \cdot \left(\mathbf {A} \times \mathbf {B} \right)=(\nabla \times \mathbf {A} )\cdot \mathbf {B} -(\nabla \times \mathbf {B} )\cdot \mathbf {A} }
∇
×
(
A
+
B
)
=
∇
×
A
+
∇
×
B
{\displaystyle \nabla \times (\mathbf {A} +\mathbf {B} )=\nabla \times \mathbf {A} +\nabla \times \mathbf {B} }
∇
×
(
ψ
A
)
=
ψ
(
∇
×
A
)
−
(
A
×
∇
)
ψ
=
ψ
(
∇
×
A
)
+
(
∇
ψ
)
×
A
{\displaystyle \nabla \times \left(\psi \mathbf {A} \right)=\psi \,(\nabla \times \mathbf {A} )-(\mathbf {A} \times \nabla )\psi =\psi \,(\nabla \times \mathbf {A} )+(\nabla \psi )\times \mathbf {A} }
∇
×
(
ψ
∇
ϕ
)
=
∇
ψ
×
∇
ϕ
{\displaystyle \nabla \times \left(\psi \nabla \phi \right)=\nabla \psi \times \nabla \phi }
∇
×
(
A
×
B
)
=
A
(
∇
⋅
B
)
−
B
(
∇
⋅
A
)
+
(
B
⋅
∇
)
A
−
(
A
⋅
∇
)
B
{\displaystyle \nabla \times \left(\mathbf {A} \times \mathbf {B} \right)=\mathbf {A} \left(\nabla \cdot \mathbf {B} \right)-\mathbf {B} \left(\nabla \cdot \mathbf {A} \right)+\left(\mathbf {B} \cdot \nabla \right)\mathbf {A} -\left(\mathbf {A} \cdot \nabla \right)\mathbf {B} }
[5] Vector-dot-Del Operator [ sửa ]
(
A
⋅
∇
)
B
=
1
2
[
∇
(
A
⋅
B
)
−
∇
×
(
A
×
B
)
−
B
×
(
∇
×
A
)
−
A
×
(
∇
×
B
)
−
B
(
∇
⋅
A
)
+
A
(
∇
⋅
B
)
]
{\displaystyle (\mathbf {A} \cdot \nabla )\mathbf {B} ={\frac {1}{2}}{\bigg [}\nabla (\mathbf {A} \cdot \mathbf {B} )-\nabla \times (\mathbf {A} \times \mathbf {B} )-\mathbf {B} \times (\nabla \times \mathbf {A} )-\mathbf {A} \times (\nabla \times \mathbf {B} )-\mathbf {B} (\nabla \cdot \mathbf {A} )+\mathbf {A} (\nabla \cdot \mathbf {B} ){\bigg ]}}
[6]
(
A
⋅
∇
)
A
=
1
2
∇
|
A
|
2
−
A
×
(
∇
×
A
)
=
1
2
∇
|
A
|
2
+
(
∇
×
A
)
×
A
{\displaystyle (\mathbf {A} \cdot \nabla )\mathbf {A} ={\frac {1}{2}}\nabla |\mathbf {A} |^{2}-\mathbf {A} \times (\nabla \times \mathbf {A} )={\frac {1}{2}}\nabla |\mathbf {A} |^{2}+(\nabla \times \mathbf {A} )\times \mathbf {A} }
Second derivatives [ sửa ]
∇
⋅
(
∇
×
A
)
=
0
{\displaystyle \nabla \cdot (\nabla \times \mathbf {A} )=0}
∇
×
(
∇
ψ
)
=
0
{\displaystyle \nabla \times (\nabla \psi )=\mathbf {0} }
∇
⋅
(
∇
ψ
)
=
∇
2
ψ
{\displaystyle \nabla \cdot (\nabla \psi )=\nabla ^{2}\psi }
scalar Laplacian )
∇
(
∇
⋅
A
)
−
∇
×
(
∇
×
A
)
=
∇
2
A
{\displaystyle \nabla \left(\nabla \cdot \mathbf {A} \right)-\nabla \times \left(\nabla \times \mathbf {A} \right)=\nabla ^{2}\mathbf {A} }
vector Laplacian )
∇
⋅
(
ϕ
∇
ψ
)
=
ϕ
∇
2
ψ
+
∇
ϕ
⋅
∇
ψ
{\displaystyle \nabla \cdot (\phi \nabla \psi )=\phi \nabla ^{2}\psi +\nabla \phi \cdot \nabla \psi }
ψ
∇
2
ϕ
−
ϕ
∇
2
ψ
=
∇
⋅
(
ψ
∇
ϕ
−
ϕ
∇
ψ
)
{\displaystyle \psi \nabla ^{2}\phi -\phi \nabla ^{2}\psi =\nabla \cdot \left(\psi \nabla \phi -\phi \nabla \psi \right)}
∇
2
(
ϕ
ψ
)
=
ϕ
∇
2
ψ
+
2
(
∇
ϕ
)
⋅
(
∇
ψ
)
+
(
∇
2
ϕ
)
ψ
{\displaystyle \nabla ^{2}(\phi \psi )=\phi \nabla ^{2}\psi +2(\nabla \phi )\cdot (\nabla \psi )+\left(\nabla ^{2}\phi \right)\psi }
∇
2
(
ψ
A
)
=
A
∇
2
ψ
+
2
(
∇
ψ
⋅
∇
)
A
+
ψ
∇
2
A
{\displaystyle \nabla ^{2}(\psi \mathbf {A} )=\mathbf {A} \nabla ^{2}\psi +2(\nabla \psi \cdot \nabla )\mathbf {A} +\psi \nabla ^{2}\mathbf {A} }
∇
2
(
A
⋅
B
)
=
A
⋅
∇
2
B
−
B
⋅
∇
2
A
+
2
∇
⋅
(
(
B
⋅
∇
)
A
+
B
×
(
∇
×
A
)
)
{\displaystyle \nabla ^{2}(\mathbf {A} \cdot \mathbf {B} )=\mathbf {A} \cdot \nabla ^{2}\mathbf {B} -\mathbf {B} \cdot \nabla ^{2}\!\mathbf {A} +2\nabla \cdot ((\mathbf {B} \cdot \nabla )\mathbf {A} +\mathbf {B} \times (\nabla \times \mathbf {A} ))}
Green's vector identity )Third derivatives [ sửa ]
∇
2
(
∇
ψ
)
=
∇
(
∇
⋅
(
∇
ψ
)
)
=
∇
(
∇
2
ψ
)
{\displaystyle \nabla ^{2}(\nabla \psi )=\nabla (\nabla \cdot (\nabla \psi ))=\nabla \left(\nabla ^{2}\psi \right)}
∇
2
(
∇
⋅
A
)
=
∇
⋅
(
∇
(
∇
⋅
A
)
)
=
∇
⋅
(
∇
2
A
)
{\displaystyle \nabla ^{2}(\nabla \cdot \mathbf {A} )=\nabla \cdot (\nabla (\nabla \cdot \mathbf {A} ))=\nabla \cdot \left(\nabla ^{2}\mathbf {A} \right)}
∇
2
(
∇
×
A
)
=
−
∇
×
(
∇
×
(
∇
×
A
)
)
=
∇
×
(
∇
2
A
)
{\displaystyle \nabla ^{2}(\nabla \times \mathbf {A} )=-\nabla \times (\nabla \times (\nabla \times \mathbf {A} ))=\nabla \times \left(\nabla ^{2}\mathbf {A} \right)}
Integration [ sửa ] Below, the curly symbol ∂ means "boundary of " a surface or solid.
Surface–volume integrals [ sửa ] In the following surface–volume integral theorems, V denotes a three-dimensional volume with a corresponding two-dimensional boundary S = ∂V (a closed surface ):
Curve–surface integrals [ sửa ] In the following curve–surface integral theorems, S denotes a 2d open surface with a corresponding 1d boundary C = ∂S (a closed curve ):
∮
∂
S
A
⋅
d
ℓ
=
∬
S
(
∇
×
A
)
⋅
d
S
{\displaystyle \oint _{\partial S}\mathbf {A} \cdot d{\boldsymbol {\ell }}\ =\ \iint _{S}\left(\nabla \times \mathbf {A} \right)\cdot d\mathbf {S} }
Stokes' theorem )
∮
∂
S
ψ
d
ℓ
=
−
∬
S
∇
ψ
×
d
S
{\displaystyle \oint _{\partial S}\psi \,d{\boldsymbol {\ell }}\ =\ -\iint _{S}\nabla \psi \times d\mathbf {S} }
∮
∂
S
A
×
d
ℓ
=
−
∬
S
(
∇
A
−
(
∇
⋅
A
)
1
)
⋅
d
S
=
−
∬
S
(
d
S
×
∇
)
×
A
{\displaystyle \oint _{\partial S}\mathbf {A} \times d{\boldsymbol {\ell }}\ =\ -\iint _{S}\left(\nabla \mathbf {A} -(\nabla \cdot \mathbf {A} )\mathbf {1} \right)\cdot d\mathbf {S} \ =\ -\iint _{S}\left(d\mathbf {S} \times \nabla \right)\times \mathbf {A} }
Integration around a closed curve in the clockwise sense is the negative of the same line integral in the counterclockwise sense (analogous to interchanging the limits in a definite integral ):
Bản mẫu:Block indent
Endpoint-curve integrals [ sửa ] In the following endpoint–curve integral theorems, P denotes a 1d open path with signed 0d boundary points
q
−
p
=
∂
P
{\displaystyle \mathbf {q} -\mathbf {p} =\partial P}
P is from
p
{\displaystyle \mathbf {p} }
q
{\displaystyle \mathbf {q} }
ψ
|
∂
P
=
ψ
(
q
)
−
ψ
(
p
)
=
∫
P
∇
ψ
⋅
d
ℓ
{\displaystyle \psi |_{\partial P}=\psi (\mathbf {q} )-\psi (\mathbf {p} )=\int _{P}\nabla \psi \cdot d{\boldsymbol {\ell }}}
gradient theorem )
A
|
∂
P
=
A
(
q
)
−
A
(
p
)
=
∫
P
(
d
ℓ
⋅
∇
)
A
{\displaystyle \mathbf {A} |_{\partial P}=\mathbf {A} (\mathbf {q} )-\mathbf {A} (\mathbf {p} )=\int _{P}\left(d{\boldsymbol {\ell }}\cdot \nabla \right)\mathbf {A} }
Ma trận [ sửa ] Trong toán học , ma trận là một mảng chữ nhật – các số , ký hiệu , hoặc biểu thức , sắp xếp theo hàng và cột – mà mỗi ma trận tuân theo những quy tắc định trước. Từng ô trong ma trận được gọi là các phần tử hoặc mục . Ví dụ một ma trận có 2 hàng và 3 cột.
[
1
9
−
13
20
5
−
6
]
.
{\displaystyle {\begin{bmatrix}1&9&-13\\20&5&-6\end{bmatrix}}.}
Kích thước hay cỡ của ma trận được định nghĩa bằng số lượng hàng và cột. Một ma trận m hàng và n cột được gọi là ma trận m × n hoặc ma trận m -nhân-n , trong khi m và n được gọi là chiều của nó. Ví dụ, ma trận A ở trên là ma trận 3 × 2.
Ma trận chỉ có một hàng gọi là vectơ hàng vectơ cột ma trận vuông ma trận vô hạn ma trận rỗng
Tên gọi
Độ lớn
Ví dụ
Miêu tả
Vectơ hàng
1 × n
[
3
7
2
]
{\displaystyle {\begin{bmatrix}3&7&2\end{bmatrix}}}
Ma trận có một hàng, được dùng để biểu diễn một vectơ
Vectơ cột
n × 1
[
4
1
8
]
{\displaystyle {\begin{bmatrix}4\\1\\8\end{bmatrix}}}
Ma trận có một cột, được dùng để biểu diễn một vectơ
Ma trận vuông n × n
[
9
13
5
1
11
7
2
6
3
]
{\displaystyle {\begin{bmatrix}9&13&5\\1&11&7\\2&6&3\end{bmatrix}}}
Ma trận có cùng số hàng và số cột, nó được sử dụng để biểu diễn phép biến đổi tuyến tính từ một không gian vec tơ vào chính nó, như phép phản xạ, phép quay hoặc ánh xạ cắt.
Ký hiệu [ sửa ] Mỗi phần tử của một ma trận thường được ký hiệu bằng một biến với hai chỉ số ở dưới. Ví dụ, a2,1 biểu diễn phần tử ở hàng thứ hai và cột thứ nhất của ma trận A . Ma trận thường được viết trong dấu ngoặc vuông:
A
=
[
a
11
a
12
⋯
a
1
n
a
21
a
22
⋯
a
2
n
⋮
⋮
⋱
⋮
a
m
1
a
m
2
⋯
a
m
n
]
.
{\displaystyle \mathbf {A} ={\begin{bmatrix}a_{11}&a_{12}&\cdots &a_{1n}\\a_{21}&a_{22}&\cdots &a_{2n}\\\vdots &\vdots &\ddots &\vdots \\a_{m1}&a_{m2}&\cdots &a_{mn}\end{bmatrix}}.}
Một cách ký hiệu khác là sử dụng dấu ngoặc đơn lớn thay cho dấu ngoặc vuông:
A
=
(
a
11
a
12
⋯
a
1
n
a
21
a
22
⋯
a
2
n
⋮
⋮
⋱
⋮
a
m
1
a
m
2
⋯
a
m
n
)
=
(
a
i
j
)
∈
R
m
×
n
.
\mathbf {A} =\left({\begin{array}{rrrr}a_{11}&a_{12}&\cdots &a_{1n}\\a_{21}&a_{22}&\cdots &a_{2n}\\\vdots &\vdots &\ddots &\vdots \\a_{m1}&a_{m2}&\cdots &a_{mn}\end{array}}\right)=\left(a_{ij}\right)\in \mathbb {R} ^{m\times n}.
Phép toán ma trận [ sửa ] Những tính chất tương tự đối với số thực có thể mở rộng ra đối với phép toán ma trận. Cộng hai ma trận có tính chất giao hoán , hay tổng của các ma trận không phụ thuộc vào thứ tự của phép tính:
A + B = B + A .(A + B ) + C = A + (B + C ) Phép chuyển vị có thể kết hợp với phép nhân vô hướng, cộng ma trận và nhân ma trận.
(c A )T = c (A T )
(A + B )T = A T + B T
(A T )T = A
(AB )T =B T A T
Phép toán
Định nghĩa
Ví dụ
Cộng hai ma trận
Tổng A +B của hai ma trận cùng kích thước m -x-n A và B được một ma trận cùng kích thước với phần tử trong vị trí tương ứng bằng tổng của hai phần tử tương ứng của mỗi ma trận:
(A + B )i ,j A i ,j B i ,j i ≤ m và 1 ≤ j ≤ n .
[
1
3
1
1
0
0
]
+
[
0
0
5
7
5
0
]
=
[
1
+
0
3
+
0
1
+
5
1
+
7
0
+
5
0
+
0
]
=
[
1
3
6
8
5
0
]
{\displaystyle {\begin{bmatrix}1&3&1\\1&0&0\end{bmatrix}}+{\begin{bmatrix}0&0&5\\7&5&0\end{bmatrix}}={\begin{bmatrix}1+0&3+0&1+5\\1+7&0+5&0+0\end{bmatrix}}={\begin{bmatrix}1&3&6\\8&5&0\end{bmatrix}}}
Nhân (vô hướng) một số với ma trận
Tích c A của số c (cũng được gọi là vô hướng trong đại số trừu tượng ) với ma trận A được thực hiện bằng cách nhân mỗi phần tử của A với c :
(c A )i ,j c • A i ,j Phép toán này được gọi là nhân vô hướng , nhưng không nên nhầm lẫn với khái niệm "tích vô hướng " hay "tích trong".
2
⋅
[
1
8
−
3
4
−
2
5
]
=
[
2
⋅
1
2
⋅
8
2
⋅
−
3
2
⋅
4
2
⋅
−
2
2
⋅
5
]
=
[
2
16
−
6
8
−
4
10
]
{\displaystyle 2\cdot {\begin{bmatrix}1&8&-3\\4&-2&5\end{bmatrix}}={\begin{bmatrix}2\cdot 1&2\cdot 8&2\cdot -3\\2\cdot 4&2\cdot -2&2\cdot 5\end{bmatrix}}={\begin{bmatrix}2&16&-6\\8&-4&10\end{bmatrix}}}
Chuyển vị
Chuyển vị của ma trận m -x-n A là ma trận n -x-m A T (cũng còn ký hiệu là A tr hay t A ) tạo ra bằng cách chuyển hàng thành cột và cột thành hàng:
(A T )i ,j A j ,i
[
1
2
3
0
−
6
7
]
T
=
[
1
0
2
−
6
3
7
]
{\displaystyle {\begin{bmatrix}1&2&3\\0&-6&7\end{bmatrix}}^{\mathrm {T} }={\begin{bmatrix}1&0\\2&-6\\3&7\end{bmatrix}}}
Tập hợp [ sửa ] Xắp sếp [ sửa ] Sếp đặt một số lượng vật vào vị trí nhứt định . Thí dụ như xắp sếp các ký tự từ a-z để tạo ra các chữ có 2 tự, 3 tự cho đến n tự . Xắp sếp các con số từ 0-9 để tạo ra các số có 1 con số , các số có 2 con số, các số có n con số
Thí dụ [ sửa ] Dùng các con số 0-9 để tạo ra số có 1 con số , có 10 xắp sếp ; số có 2 con số , có 10 x 9 = 90 xắp sếp ; số có 3 con số , có 10 x 9 x 8 = 720 xắp sếp
Từ đó, ta có
P
(
m
,
n
)
=
m
!
(
m
−
n
)
!
{\displaystyle P(m,n)={\frac {m!}{(m-n)!}}}
P
(
10
,
1
)
=
10
!
(
10
−
1
)
!
=
10987654321
987654321
=
10
{\displaystyle P(10,1)={\frac {10!}{(10-1)!}}={\frac {10987654321}{987654321}}=10}
Công thức [ sửa ]
P
(
m
,
m
)
=
m
!
{\displaystyle P(m,m)=m!}
P
(
m
,
n
1
,
n
2
,
n
n
)
=
m
!
n
1
!
n
2
!
n
n
!
{\displaystyle P(m,n_{1},n_{2},n_{n})={\frac {m!}{n_{1}!n_{2}!n_{n}!}}}
Kết hợp [ sửa ]
▲ Feynman, R. P.; Leighton, R. B.; Sands, M. (1964). The Feynman Lectures on Physics . Addison-Wesley. Vol II, p. 27–4. ISBN 0-8053-9049-9 . ▲ Bản mẫu:Cite arXiv ▲ Doran, C.; Lasenby, A. (2003). Geometric algebra for physicists . Cambridge University Press. tr. 169. ISBN 978-0-521-71595-9 . ▲ Kelly, P. (2013). "Chapter 1.14 Tensor Calculus 1: Tensor Fields ". Mechanics Lecture Notes Part III: Foundations of Continuum Mechanics . http://homepages.engineering.auckland.ac.nz/~pkel015/SolidMechanicsBooks/Part_III/Chapter_1_Vectors_Tensors/Vectors_Tensors_14_Tensor_Calculus.pdf . Truy cập 7 December 2017 . ▲ "lecture15.pdf" . https://www2.ph.ed.ac.uk/~mevans/mp2h/VTF/lecture15.pdf . ▲ Kuo, Kenneth K.; Acharya, Ragini (2012). Applications of turbulent and multi-phase combustion doi :10.1002/9781118127575.app1 . ISBN 9781118127575 . https://onlinelibrary.wiley.com/doi/pdf/10.1002/9781118127575.app1 . Truy cập 19 April 2020 .
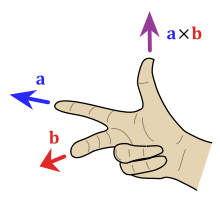
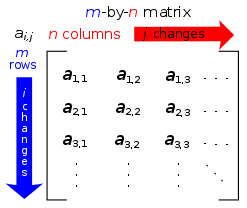
![{\displaystyle {\sqrt[{m}]{\sqrt[{n}]{a}}}={\sqrt[{mn}]{a}}=a^{\frac {1}{mn}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/83064172516d2185efbd65172ed7cc9d3e701284)
![{\displaystyle {\sqrt[{n}]{\frac {a}{b}}}={\frac {\sqrt[{n}]{a}}{\sqrt[{n}]{b}}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/372ddb7d13541806e35a6053ba614df98a87b655)
![{\displaystyle a+(a+d)+(a+2d)+...+[a+(n-1)d]=\sum _{k=0}^{\infty }[a+(n-1)d]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/85c5b6f1d86ff3f156fd65ff5857f5e95f89c10e)
![{\displaystyle \sum _{k=0}^{\infty }[a+(n-1)d]=a+(a+d)+(a+2d)+...+[a+(n-1)d]={\frac {n}{2}}(2a+(n-1)d)}](https://wikimedia.org/api/rest_v1/media/math/render/svg/bc2a5db1feeaf9814ba2e7c2618c255f45f299a8)
![{\displaystyle S=a+(a+d)+(a+2d)+...+[a+(n-1)d]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/63777c0be192f9fe36871fbf7d21970aaed89899)
![{\displaystyle S=[a+(n-1)d]+...+(n-1)d]+a}](https://wikimedia.org/api/rest_v1/media/math/render/svg/5c376b47de6ed1cfc662cad50bbc979688886f30)
![{\displaystyle 2S=[2a+(n-1)d]n}](https://wikimedia.org/api/rest_v1/media/math/render/svg/f47f5f57718103fa0610bc7149959105b3a09039)
![{\displaystyle S=[2a+(n-1)d]{\frac {n}{2}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/220eac7734e9963f251e2cac7519edcaf6af5420)
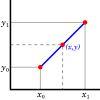
![{\displaystyle s=\Delta xy+\Delta x{\frac {\Delta y}{2}}=\Delta x[y+{\frac {\Delta y}{2}}]=\Delta x[f(x)+{\frac {\Delta f(x)}{2}}]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/97c1e8dd2152f12fb2ce22cb9e0aa68d4796d786)
![{\displaystyle s=\Delta x[f(x)+{\frac {\Delta f(x)}{2}}]={\frac {\Delta x}{2}}[2f(x)+f(x+\Delta x)-f(x)]={\frac {\Delta x}{2}}[f(x)+f(x+\Delta x)]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/26da47a72c8fdda1d4c93ae221c06fe1ee8d89a5)
<
![{\displaystyle [{\vec {a}},{\vec {b}}]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/008d8868e90625dc41200b2396382596923b8d9c)
![{\displaystyle [{\vec {n_{1}}},{\vec {n_{2}}}]=({\begin{vmatrix}B_{1}&C_{1}\\B_{2}&C_{2}\end{vmatrix}},{\begin{vmatrix}C_{1}&A_{1}\\C_{2}&A_{2}\end{vmatrix}},{\begin{vmatrix}A_{1}&B_{1}\\A_{2}&B_{2}\end{vmatrix}})}](https://wikimedia.org/api/rest_v1/media/math/render/svg/14cf919fe1c939d7c84b9d19d5bc78bd08474340)
![{\displaystyle S=\left\vert [{\vec {AB}};{\vec {AD}}]\right\vert =AB.AD.sin(A)}](https://wikimedia.org/api/rest_v1/media/math/render/svg/760fdf915998c29a66e0f1db274670f50bc32c72)
![{\displaystyle V=\left\vert [{\vec {AB}};{\vec {AD}}]\cdot {\vec {AA'}}\right\vert }](https://wikimedia.org/api/rest_v1/media/math/render/svg/59ee277ddb227df9df6bc11dc54f1b1d3c9c7efe)
![{\displaystyle [{\vec {u}};{\vec {v}}]={\vec {0}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/902f2c5070ec2906fba5cdfebc75db816024ab33)
![{\displaystyle [{\vec {u}};{\vec {v}}].{\vec {w}}=0}](https://wikimedia.org/api/rest_v1/media/math/render/svg/29ff4069898c2e3c87418e03aeb2464f9f0777a4)
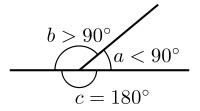
![{\displaystyle s=\Delta x[f(x)+{\frac {\Delta f(x)}{2}}]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/6e2b2dec85c8fc14028ca9525826bd5e7ecf1559)
![{\displaystyle {\begin{aligned}\operatorname {curl} \mathbf {F} &=\nabla \times \mathbf {F} ={\begin{pmatrix}\displaystyle {\frac {\partial }{\partial x}},\ {\frac {\partial }{\partial y}},\ {\frac {\partial }{\partial z}}\end{pmatrix}}\times {\begin{pmatrix}F_{x},\ F_{y},\ F_{z}\end{pmatrix}}={\begin{vmatrix}\mathbf {i} &\mathbf {j} &\mathbf {k} \\{\frac {\partial }{\partial x}}&{\frac {\partial }{\partial y}}&{\frac {\partial }{\partial z}}\\F_{x}&F_{y}&F_{z}\end{vmatrix}}\\[1em]&=\left({\frac {\partial F_{z}}{\partial y}}-{\frac {\partial F_{y}}{\partial z}}\right)\mathbf {i} +\left({\frac {\partial F_{x}}{\partial z}}-{\frac {\partial F_{z}}{\partial x}}\right)\mathbf {j} +\left({\frac {\partial F_{y}}{\partial x}}-{\frac {\partial F_{x}}{\partial y}}\right)\mathbf {k} \end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/8579a43f51c457ddcad8e559206bf6e1696b1419)
![{\displaystyle {\begin{aligned}\nabla \left({\frac {\psi }{\phi }}\right)&={\frac {\phi \,\nabla \psi -\psi \,\nabla \phi }{\phi ^{2}}}\\[1em]\nabla \left({\frac {\mathbf {A} }{\phi }}\right)&={\frac {\phi \,\nabla \mathbf {A} -\nabla \phi \otimes \mathbf {A} }{\phi ^{2}}}\\[1em]\nabla \cdot \left({\frac {\mathbf {A} }{\phi }}\right)&={\frac {\phi \,\nabla {\cdot }\mathbf {A} -\nabla \!\phi \cdot \mathbf {A} }{\phi ^{2}}}\\[1em]\nabla \times \left({\frac {\mathbf {A} }{\phi }}\right)&={\frac {\phi \,\nabla {\times }\mathbf {A} -\nabla \!\phi \,{\times }\,\mathbf {A} }{\phi ^{2}}}\\[1em]\nabla ^{2}\left({\frac {\psi }{\phi }}\right)&={\frac {\phi \,\nabla ^{2\!}\psi -2\,\phi \,\nabla \!\left({\frac {\psi }{\phi }}\right)\cdot \!\nabla \phi -\psi \,\nabla ^{2\!}\phi }{\phi ^{2}}}\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/93a497c998c46163f4eb8615f7cf7c6793c2f475)
![{\displaystyle {\begin{aligned}\nabla \cdot (\mathbf {A} \times \mathbf {B} )&\ =\ (\nabla {\times }\mathbf {A} )\cdot \mathbf {B} \,-\,\mathbf {A} \cdot (\nabla {\times }\mathbf {B} )\\[5pt]\nabla \times (\mathbf {A} \times \mathbf {B} )&\ =\ \mathbf {A} (\nabla {\cdot }\mathbf {B} )\,-\,\mathbf {B} (\nabla {\cdot }\mathbf {A} )\,+\,(\mathbf {B} {\cdot }\nabla )\mathbf {A} \,-\,(\mathbf {A} {\cdot }\nabla )\mathbf {B} \\[2pt]&\ =\ \mathbf {A} (\nabla {\cdot }\mathbf {B} )\,+\,(\mathbf {B} {\cdot }\nabla )\mathbf {A} \,-\,(\mathbf {B} (\nabla {\cdot }\mathbf {A} )\,+\,(\mathbf {A} {\cdot }\nabla )\mathbf {B} )\\[2pt]&\ =\ \nabla {\cdot }\left(\mathbf {B} \mathbf {A} ^{\textsf {T}}\right)\,-\,\nabla {\cdot }\left(\mathbf {A} \mathbf {B} ^{\textsf {T}}\right)\\[2pt]&\ =\ \nabla {\cdot }\left(\mathbf {B} \mathbf {A} ^{\textsf {T}}\,-\,\mathbf {A} \mathbf {B} ^{\textsf {T}}\right)\\[5pt]\mathbf {A} \times (\nabla \times \mathbf {B} )&\ =\ \nabla _{\mathbf {B} }(\mathbf {A} {\cdot }\mathbf {B} )\,-\,(\mathbf {A} {\cdot }\nabla )\mathbf {B} \\[2pt]&\ =\ \mathbf {A} \cdot \mathbf {J} _{\mathbf {B} }\,-\,(\mathbf {A} {\cdot }\nabla )\mathbf {B} \\[2pt]&\ =\ (\nabla \mathbf {B} )\cdot \mathbf {A} \,-\,\mathbf {A} \cdot (\nabla \mathbf {B} )\\[2pt]&\ =\ \mathbf {A} \cdot (\mathbf {J} _{\mathbf {B} }\,-\,\mathbf {J} _{\mathbf {B} }^{\textsf {T}})\\[5pt](\mathbf {A} \times \nabla )\times \mathbf {B} &\ =\ (\nabla \mathbf {B} )\cdot \mathbf {A} \,-\,\mathbf {A} (\nabla {\cdot }\mathbf {B} )\\[2pt]&\ =\ \mathbf {A} \times (\nabla \times \mathbf {B} )\,+\,\mathbf {A} {\cdot }(\nabla \mathbf {B} )\,-\,\mathbf {A} (\nabla {\cdot }\mathbf {B} )\\[5pt](\mathbf {A} \times \nabla )\cdot \mathbf {B} &\ =\ \mathbf {A} \cdot (\nabla {\times }\mathbf {B} )\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/c60da17a8d21edeef2d47f3a8806664ec3527e53)
![{\displaystyle (\mathbf {A} \cdot \nabla )\mathbf {B} ={\frac {1}{2}}{\bigg [}\nabla (\mathbf {A} \cdot \mathbf {B} )-\nabla \times (\mathbf {A} \times \mathbf {B} )-\mathbf {B} \times (\nabla \times \mathbf {A} )-\mathbf {A} \times (\nabla \times \mathbf {B} )-\mathbf {B} (\nabla \cdot \mathbf {A} )+\mathbf {A} (\nabla \cdot \mathbf {B} ){\bigg ]}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/8cd10d81d948e6fa928365812a1c43d2cb14330a)
